X 2 y 2 = 2 5 Subtract y^ {2} from both sides Subtract y 2 from both sides x^ {2}=25y^ {2} x 2 = 2 5 − y 2 Take the square root of both sides of the equation Take the square root of both sides of the equation x=\sqrt {25y^ {2}} x=\sqrt {25y^ {2}} x = 2 5 − y 2 x = − 2 5 − y 2X^2y^2=25 xy=12 then x= 2 See answers x can be 3 and 4 zahir79zahir79 Here the value of x=3 and y=4 so, 3^24^2=25Observing , it's obvious that x and y MUST be 3 and 4 Likewise x and y MUST be 3 and 4 as xy = 12 Proving this, we get> , which becomes 25 2(12) = 25 24 = 49 Since , then x y = 7 Square root of each side was taken I don't know what means but you should be able to

Solutions Manual For Beginning Algebra 7th Edition By Martin Gay By Cndps109 Issuu
X^2+y^2=25 formula
X^2+y^2=25 formula-As someone else stated, x 2 y 2 = 25 is a relationship describing a circle this link shows the generic equation and the meanings as such In this case, the center of the circle is at the point (0,0) and the radius is 25 units 1 Share Report Save level 1 5 years agoFeb 09, 16 · Explanation The center of the circle is at (0,0) and, when x = 0, the circle points are at y = − 5 and y = 5 So, the radius of the circle is r = 5 The area of a circle is given by πr2 So, substituting r = 5, one gets the answer 25π Answer link


Help With Quadratic Simultaneous Equation Gcse
So we are given 2 equations mathx^2 y^2 = 25/math mathxy = 12/math And wish to find all possible solutions for this Let us start by using the second equation and solving for y mathy = \frac{12}{x}/math Which gives mathx^2 \fr2 We can describe a point, P, in three different ways Cartesian Cylindrical Spherical Cylindrical Coordinates x = r cosθ r = √x2 y2 y = r sinθ tan θ = y/x z = z z = z Spherical CoordinatesApr 18, 15 · If x^2 2xy y^2 = 25, then (x y)^3 could be 21 Jun 17, 0221 Expert Reply 0000 Question Stats 97% (0036) correct 2% (0029) wrong based on 69 sessions Hide Show timer Statistics This question is part of GREPrepClub The Questions Vault Project If \(x^2\) 2xy \(y^2\) = 25, then \((x y)^3\) could be
(1) x 2 y 2 = 25 (2) y 3x = 13 To solve simultaneous equations algebraically we want to rearrange one of the equations to be able to substitute this in to the other equation In this example, we have squared x and y terms, which makes this equation the more complex one We therefore decide to rearrange equation (2), and as the y term here has a coefficient of 1 it is easiest to rearrangeThis video explains how to derive the area formula for a circle using integrationhttp//mathispower4ucomXy=12, (x^2y^2)=25 then what is the value of (xy)2^2;
Find the center, transverse axis, vertices, foci, and asymptotes for the hyperbolaDec 04, 07 · x^2 y^2 = 25 3y 4x = 0 you can do this graphically x^2 y^2 = 25 is a circle with center at origin and radius =5 and 3y 4x = 0 is the equation of a line y = 4x/3 the solution = intersection points of the line with the circleStart with (x−4)2 (y−2)2 = 25 Move (x−4) 2 to the right (y−2)2 = 25 − (x−4)2 Take the square root (y−2) = ± √ 25 − (x−4)2 (notice the ± "plus/minus" there can be two square roots!) Move the "−2" to the right y = 2 ± √ 25 − (x−4)2 So when


Solved Y V1 X2 1 31 2 X Y 2 25 X Y 3 1 Chegg Com



1 2 Solving Equations By The Quadrus Method Levels 1 2 3 G Day Math
$x^4 4x^2 4 (x^2 4)(x^2 4)=x^4 4x^2 4 (x^4 16) = x^4 4x^2 4 x^4 16 = 4x^2 $ 3) Solve the equation x 2 25 = 0 Solution x 2 25 = (x 5)(x 5)Nov 15, 06 · x^2y^2=25 sub y=(x!) x^2(x1)^2=25 x^2x^22x1=25 2x^22x=24 2(x^21)=24 x^21=12 x^2=13 x=/rt13 y=x1 y=rt131Stack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange


Y Mx C High Resolution Stock Photography And Images Alamy



Solve System X 2 Y 2 25 Y X 2 5 Youtube
Whenever you get two equations and two unknowns, chances are you might have to substitute in for a variable to find the solutionSep 10, 08 · There are in fact six solutions to this problem x^2 y^2 = 25 x^2 = 25 y^2 x = √(25 y^2) substitute this value of x into the other equationFree PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystep



Calculus Iii 2210 4 Final Exam Study Guide Name


Solved Y V1 X2 1 31 2 X Y 2 25 X Y 3 1 Chegg Com
Plugging into the parabola formula for x we can calculate the y coordinate y = 10 * 050 * 050 10 * 050 250 or y = Parabola, Graphing Vertex and XIntercepts Root plot for y = x 2x25 Axis of Symmetry (dashed) {x}={ 050} Vertex at {x,y} = { 050,2525} x Intercepts (Roots) Root 1 at {x,y} = {452, 000}Aug 09, 10 · The line x7y=25 cuts the circle x^2y^2=25 at two points A and B Find, (a)the coordinates of A and B (b)the equation of the perpendicular bisector of AB and show that it passes through the centre of the circle, (c)the coordinates ofA wonderfully archaic term, subtangent The subtangent is the distance between where the tangent to the curve at the point meets the xaxis, and the projection of the point on the xaxis In this case, you are helpfully given a circle centred on t


Ppt Circles In The Coordinate Plane Powerpoint Presentation Free Download Id


What Are The Equations Of Tangent Lines To The Hyperbola X2 9y2 25 That Pass Through The Point D 5 5 3 Quora
In mathematics, an implicit equation is a relation of the form R(x 1,, x n) = 0, where R is a function of several variables (often a polynomial)For example, the implicit equation of the unit circle is x 2 y 2 − 1 = 0 An implicit function is a function that is defined by an implicit equation, that relates one of the variables, considered as the value of the function, with the othersMay 17, 07 · PROB 1 x^2y^2=25 & y=x1 PROB 2 x^2y^2=25 & x^2y^2=5 Please show me how?Mar 14, 21 · Solve using the quadratic formula \(3 x^{2}6 x2=0\) Solution Begin by identifying \(a,b\), and \(c\) \(a=3 \quad b=6 \quad c=2\) Substitute these values into the quadratic formula (Equation \ref{quad}) At this point we see that \(60 = 4 \times 15\) and thus the fraction can be simplified further
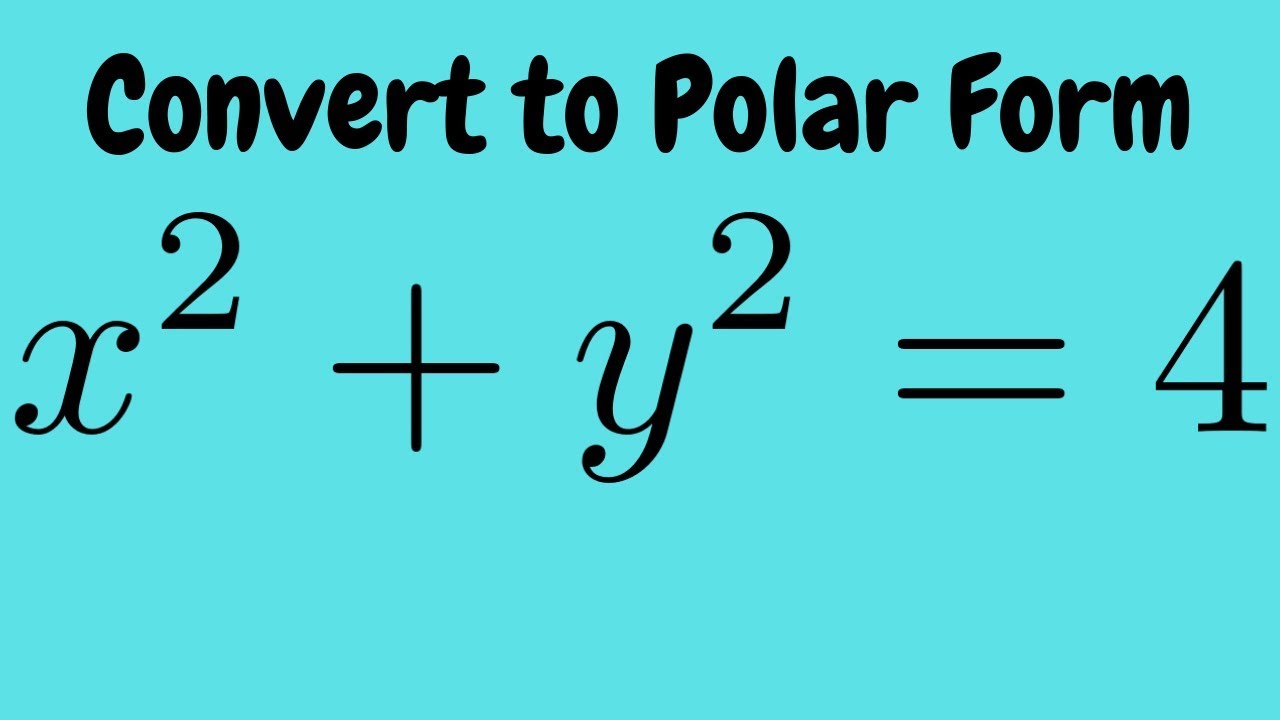


Converting The Rectangular Equation X 2 Y 2 4 Into Polar Form Youtube



Equation Of A Circle Analytical Geometry Siyavula
Find the Center and Radius x^2y^2=25 x2 y2 = 25 x 2 y 2 = 25 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2 Match the values in this circle to those of the standard formSlope is defined as the change in y divided by the change in x We note that for x=0, the value of y is 0000 and for x=00, the value of y is 00 So, for a change of 00 in x (The change in x is sometimes referred to as "RUN") we get a change of 00 0000 = 00 in yClick here👆to get an answer to your question ️ If x^2 y^2 = 25,xy = 12 , then the number of values of x is
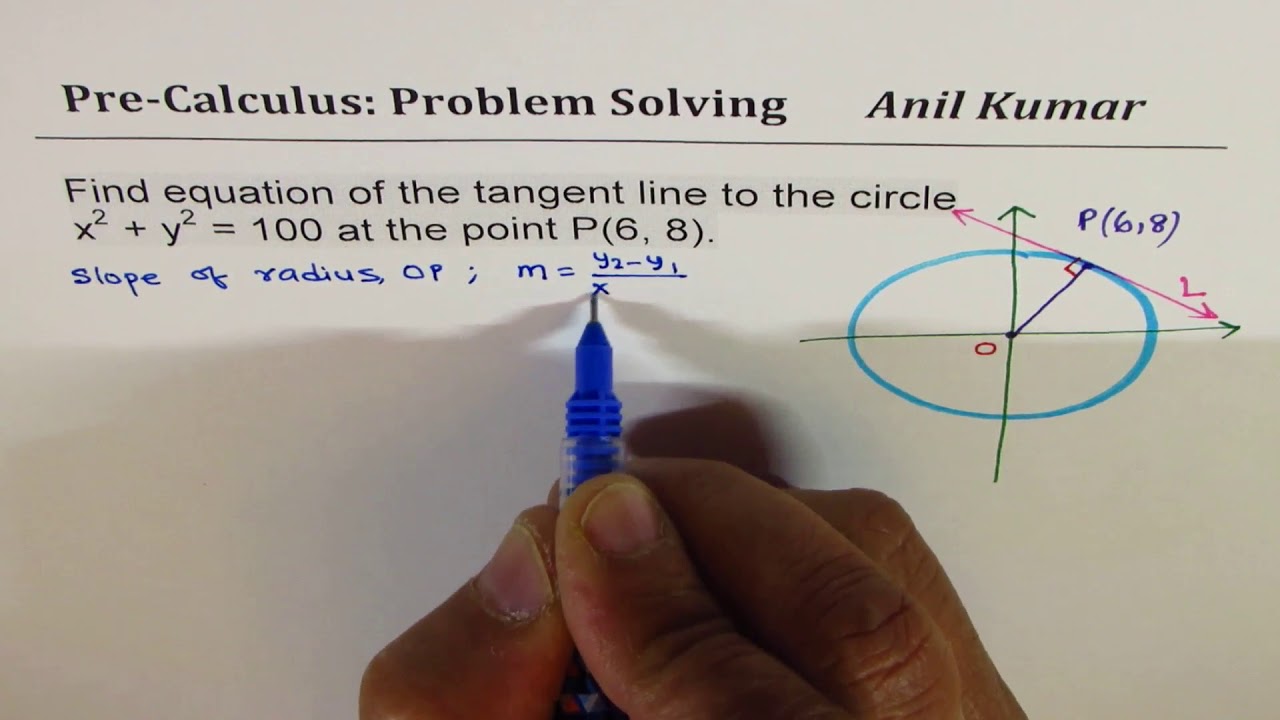


Equation Of Tangent Line To Circle X 2 Y 2 100 At Point 6 8 Youtube



If The Straight Line X 2y 1 0 Intersects The Circle X 2
Jun 23, 10 · ==> x = y^2/25 ==> y^2 = 25x Then, substitute y^2 with 25x in the equation of the circle to find the xcoordinates of intersection x^2 25x = 25 ==> x^2 25x 25 = 0 By the Quadratic Formula, x = (25 5√29)/2 and x = (25 5√29)/2 Then since y^2 = 25x, we have y^2 = 25(25 5√29)/2 ==> y^2 = (625 125√29)/2Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyFeb 12, 14 · If x^2y^2=25, find dy/dt when x=3 and dx/dt= 8 Solve the following problems by using the binomial formula a If n = 4 and p = 10 , find P(x = 3) b If n = 7 and p = 80 , find P(x = 4) Given the function f(x) = x^2 1 / x^2 9 a)find y and x intercepts b) find the first derivative c) find any critical values d) find any



How Do You Integrate Sqrt X 2 25 X Example


Ellipses
Solve algebraically the simultaneous equations x^2 y^2 = 25 and y x = 1 Let's label the equations x 2 y 2 = 25 (1) y x = 1 (2) We can use substitution to solve this simultaneous equation Let's make y the subject of the equation (2) and we get y = x 1 Using the quadratic formula factorise 2x^2 7x 11 =0 Answered by Emily AΔ = b 24ac Δ = 0 24·2·(25) Δ = 0 The delta value is higher than zero, so the equation has two solutions We use following formulas to calculate our solutionsJan 26, 07 · 1) Find the second derivative of x^2 y ^ 2 = 25 I can only find the first derivative i can't find the second 2) Find the second derivative of y = x^2 y^3 xy I actually have no clue how to find the second derviative This sort of question is going to be on a test, but my teacher didn't cover it So please explain it step by step Thank you!
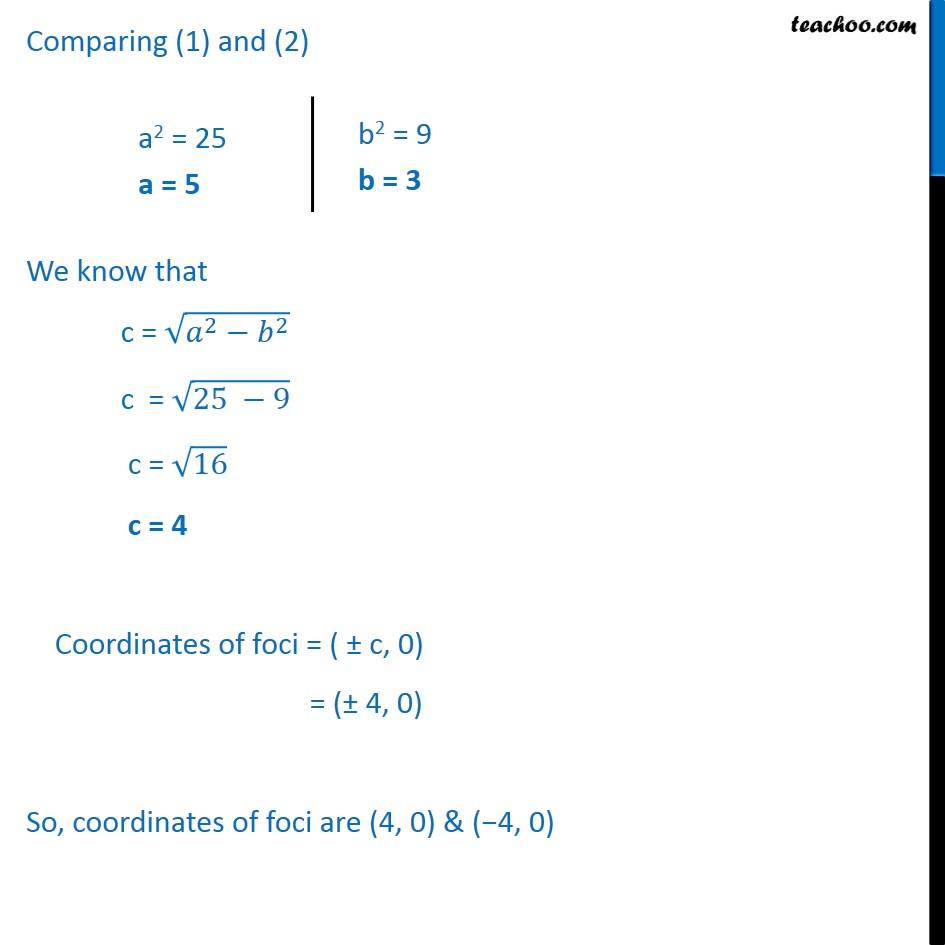


Example 9 X2 25 Y2 9 1 Find Foci Vertices Eccentricity



Math Question
(2,1) This is where i'm stuck I know how to get up to the first equationand I know how to get to the final answer from the second equation, but IThe circle of x^2 y^2 = 25 has a radius of 5 units and the center of the circle is at the point (0,0) to graph the circle you solve for y equation would be y = / sqrt (25x^2) and would look like this on the graph The equation of the radius intersecting the circle atSolution for X2y2=25 equation X2X2=25 We move all terms to the left X2X2(25)=0 We add all the numbers together, and all the variables 2X^225=0 a = 2;



The Equation Of The Tangent To The Circle X 2 Y 2 25 Passing Thr



Vertices Direction Of A Hyperbola Example 2 Video Khan Academy
Answer to A particle moves on the circle x 2 y 2 = 25 in the xyplane during a period of time represented by t 0 At the time when theMar 02, 12 · Use implicit differentiation to find the slope of the tangent line to the curve at the specified point 3(x^2 y^2)^2 = 25(x^2 y^2) ;X2 y2 = 25 x 2 y 2 = 25 , x − y = 1 x y = 1 Add y y to both sides of the equation x = 1 y x = 1 y x2 y2 = 25 x 2 y 2 = 25 Replace all occurrences of x x with 1y 1 y in each equation Tap for more steps Replace all occurrences of x x in x 2 y 2 = 25 x 2 y 2 = 25 with 1 y 1 y



Equation Of A Tangent To A Circle Analytical Geometry Siyavula
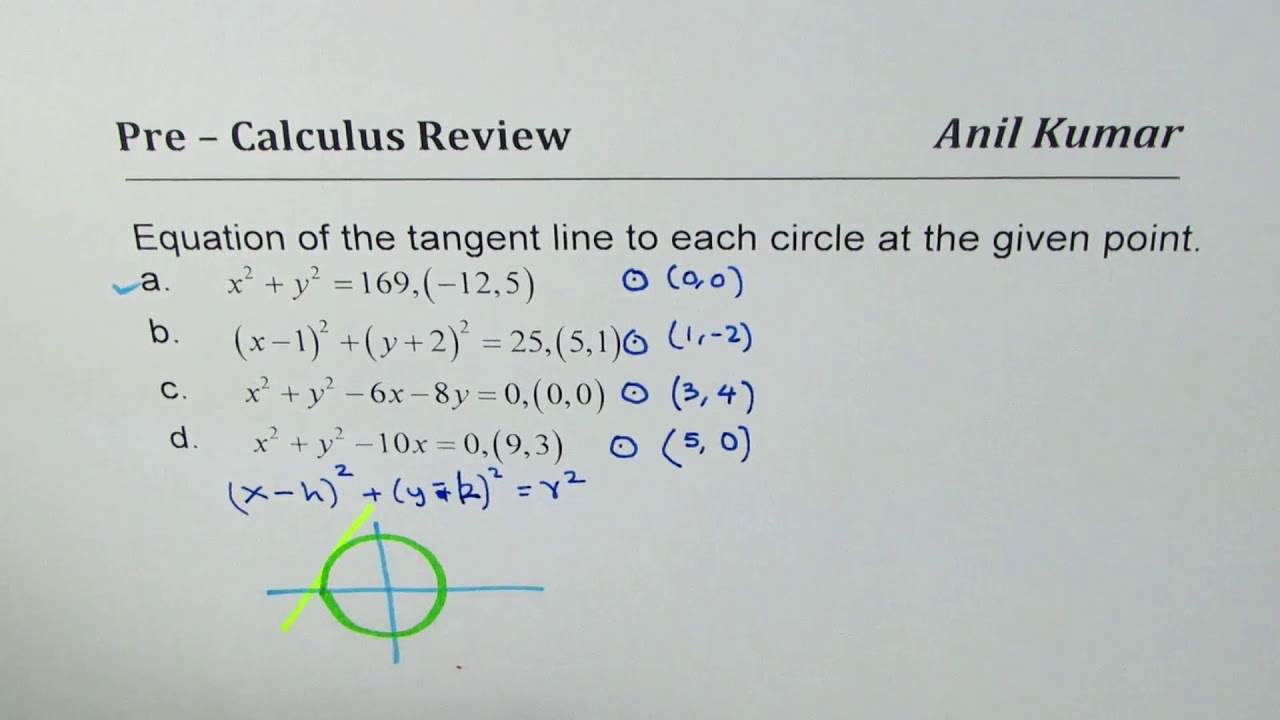


Equation Of Tangent Line On Circle X 1 2 Y 2 2 25 Youtube
Mar 12, 13 · Favorite Answer x^2 2xy y^2 = 25 factor the left side (x y) (x y) = 25 take the square root of each side x y = 5 or x y = 5 solve for y y = x 5 or y = x 5 since y > xJul 01, 18 · The general formula of a circle is given by (x− h)2 (y −k)2 = r2 where (h,k) is the centre is r is the radius Therefore, x2 y2 = 25 can also be written as (x −0)2 (y −0)2 = 52Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more



Which Graph Represents The Solution Of The System X 2 Y 2 4 X Y 1 Brainly Com
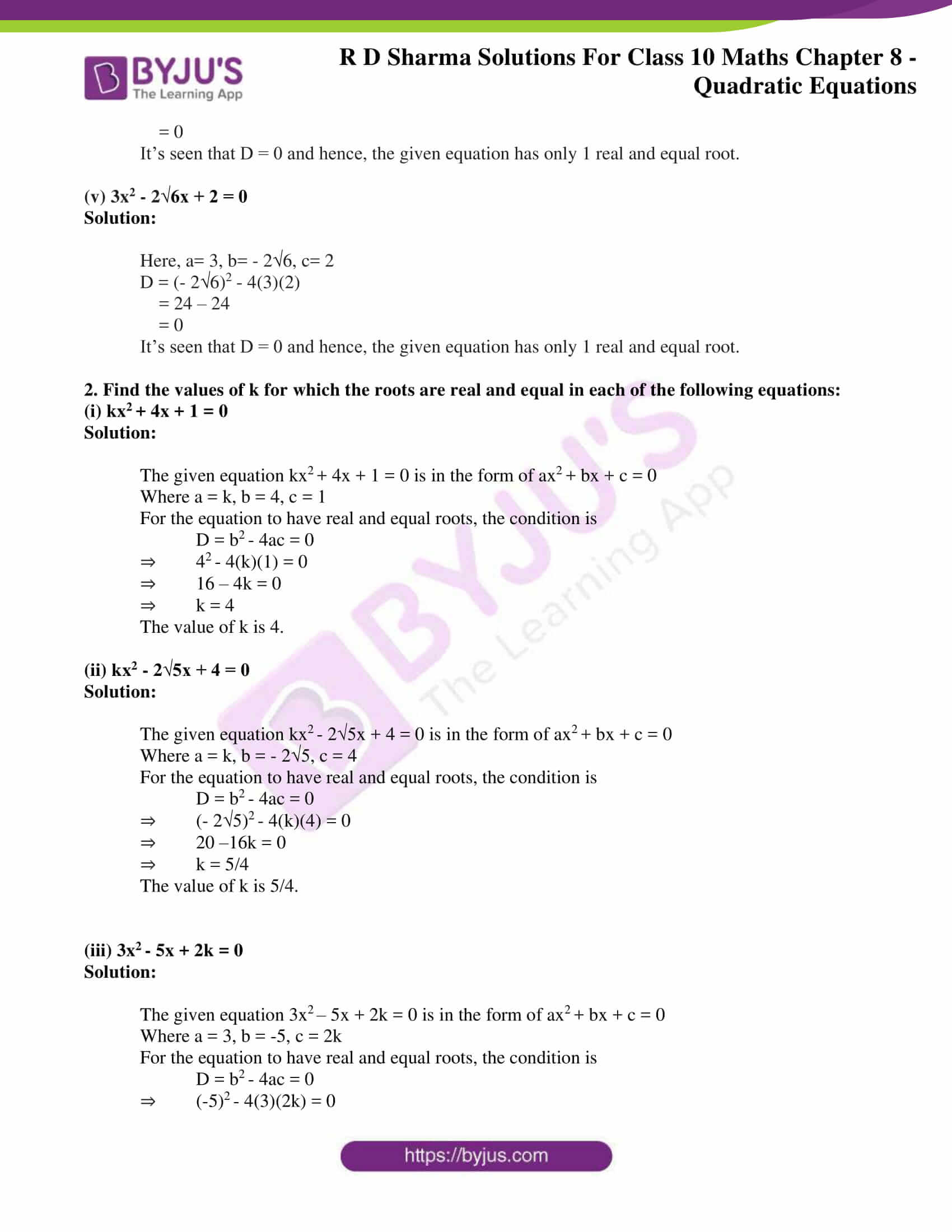


Rd Sharma Solutions For Class 10 Chapter 8 Quadratic Equations Exercise 8 6 Avail Pdf
Simple and best practice solution for x2y=25 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,Oct 18, 11 · x=0 not strictly in 1st quadrant so 4(x^2y^2)=25 and sub into equation of curve I make the point (5sqrt3/4,5/4) Another approach is using polar coordinates giving polar equation 2r^2=25cos(2theta)Identify the vertices and foci of the hyperbola with equation x 2 9 − y 2 25 = 1 x 2 9 − y 2 25 = 1 Writing Equations of Hyperbolas in Standard Form Just as with ellipses, writing the equation for a hyperbola in standard form allows us to calculate the key features its center, vertices, covertices, foci, asymptotes, and the lengths and
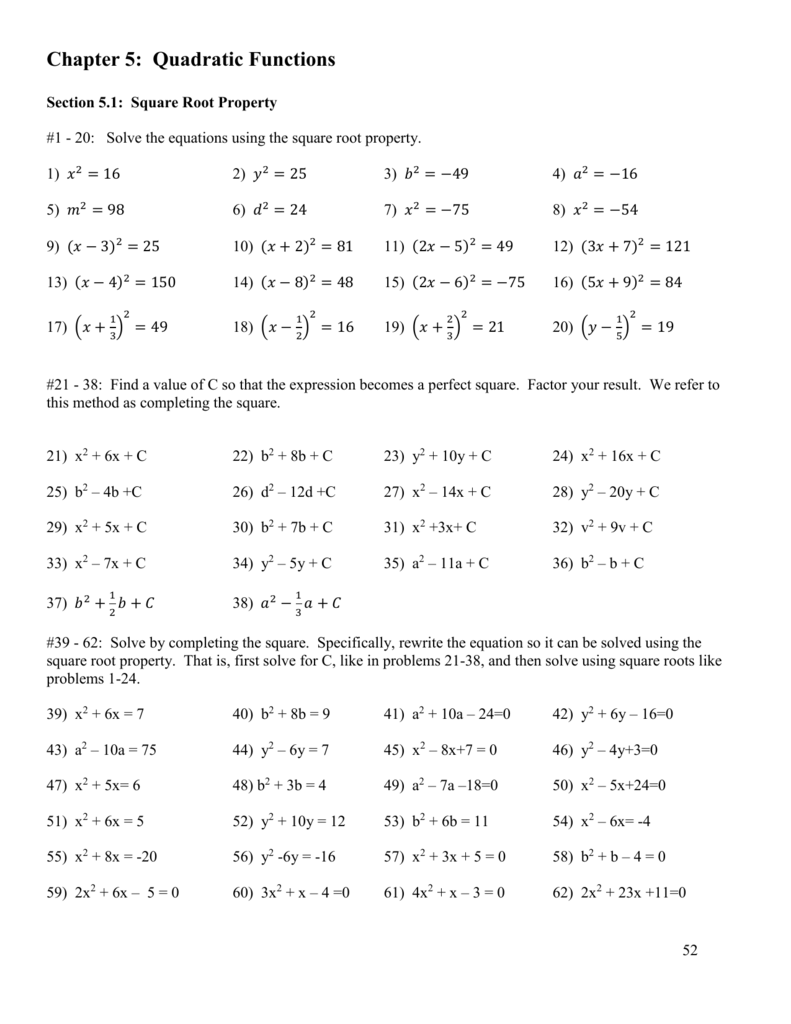


Chapter 5 Problems



Solutions Manual For Beginning Algebra 7th Edition By Martin Gay By Cndps109 Issuu
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyMay 26, · Again, this is set up to use the initial formula we gave in this section once we realize that the equation for the bottom is given by \(g\left( {x,y} \right) = 0\) and \(D\) is the disk of radius \(\sqrt 3 \) centered at the origin Also, don't forget to plug in$f(x,y)=x^2y^225=0$ $\frac{d}{dx}{f(x,y)}=2x\frac{d}{dx}{(y^2)}=2x\frac{d}{dy}{(y^2)}\frac{dy}{dx}=2x2y\frac{dy}{dx}=0$ $2x2y\frac{dy}{dx}=2x 2\sqrt{25x^2} \frac{dy}{dx}=0$



Ways To Solve Quadratic Equations Algebra I Discriminant En Equation Factoring Math Ways To Solve Quadra Glogster Edu Interactive Multimedia Posters
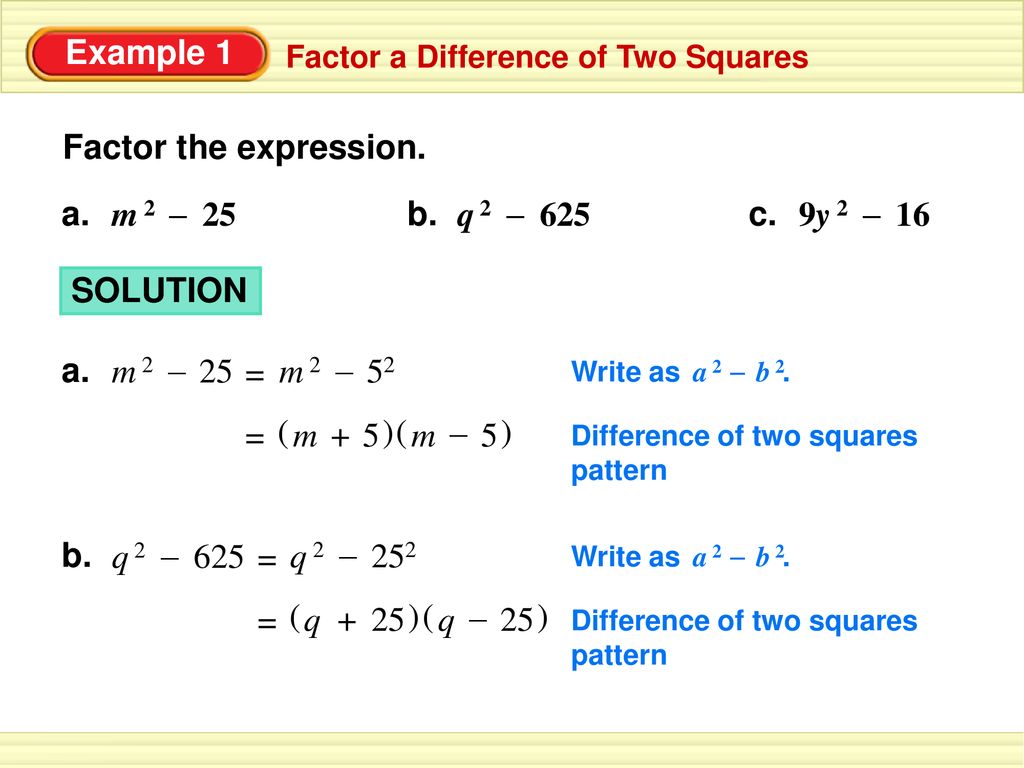


Example 1 Factor The Expression A M 2 25 B Q 2 625 C 9y 2 Ppt Download
You can put this solution on YOUR website!


How To Solve 1 5 2 25 3 325 4 4325 5 Quora


Help With Quadratic Simultaneous Equation Gcse



If X 2 Y 2 25 Then Find Dy Dx



Example 1 Factor The Expression A M 2 25 B Q 2 625 C 9y 2 Ppt Download
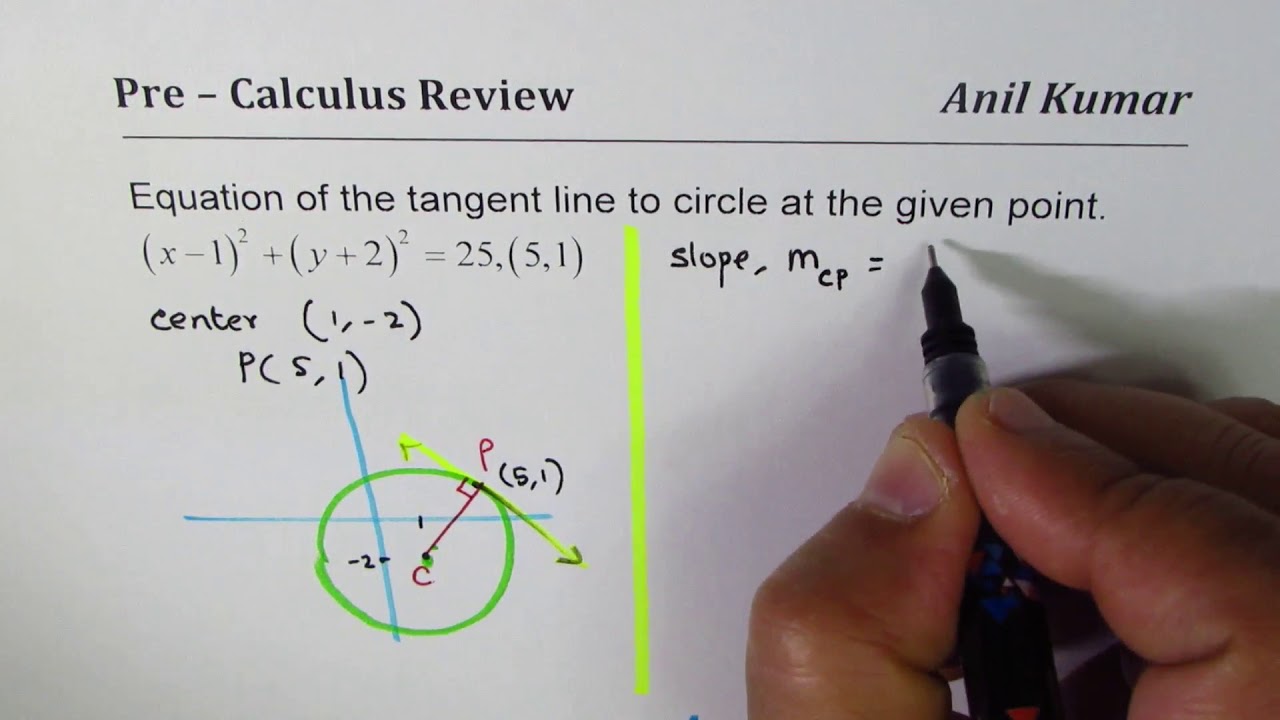


Equation Of Tangent Line On Circle X 1 2 Y 2 2 25 Youtube


Equation Of Tangent Line On Circle X 1 2 Y 2 2 25 Youtube



Then Equation Of Auxiliary Circle Of The Ellipse 16x 2 25y 2


Complete The Square Lesson Ppt Download



5 Solve The Quadratic Equation With The Square Root Property X 4 2 Quadratics Quadratic Equation Square Roots
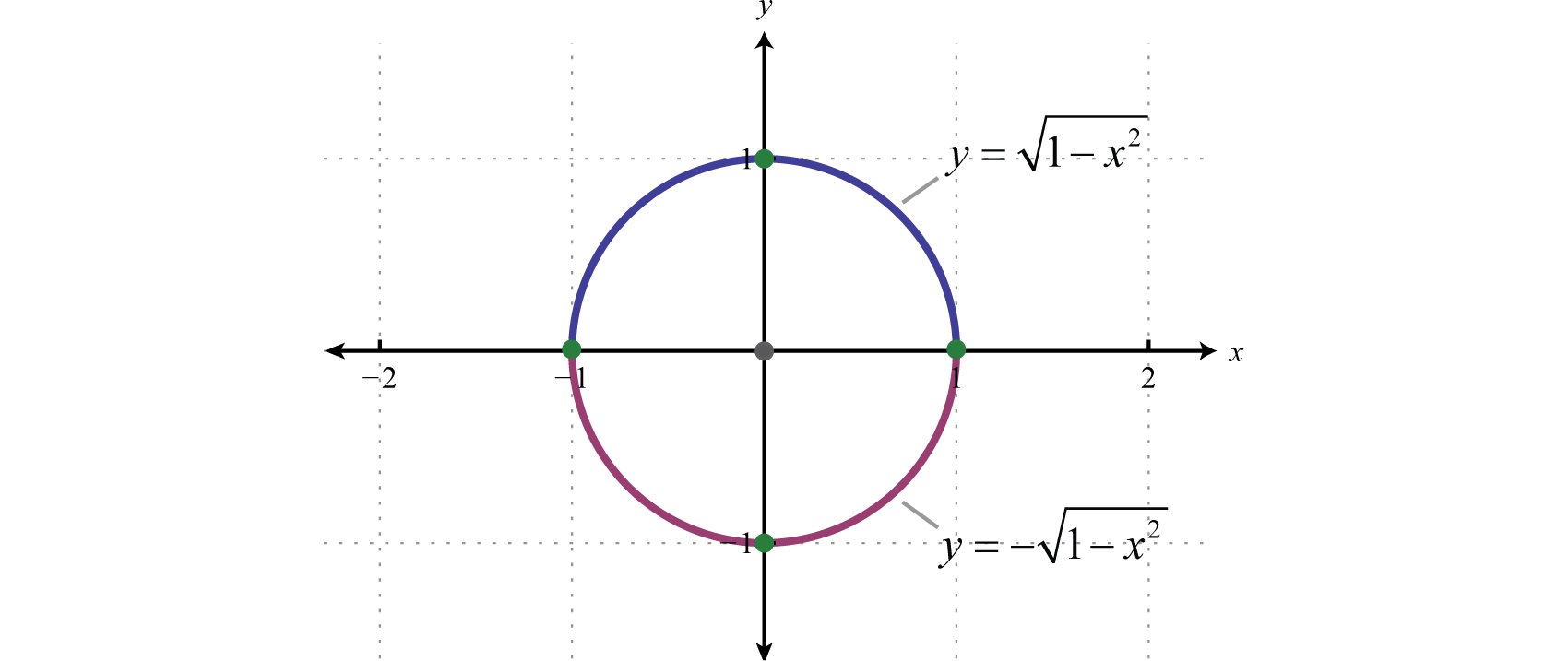


Circles



What Is The Solution Set To The Following System X Y 5 X 2 Y 2 25 A 0 5 5 0 B 0 5 5 0 C Brainly Com



Find A Vector Equation For The Tangent Line To The Curve Of Intersection Of The Cylinders X2 Y2 25 And Y2 Z2 At The Point 3 4 2 Homework Help And Answers Slader
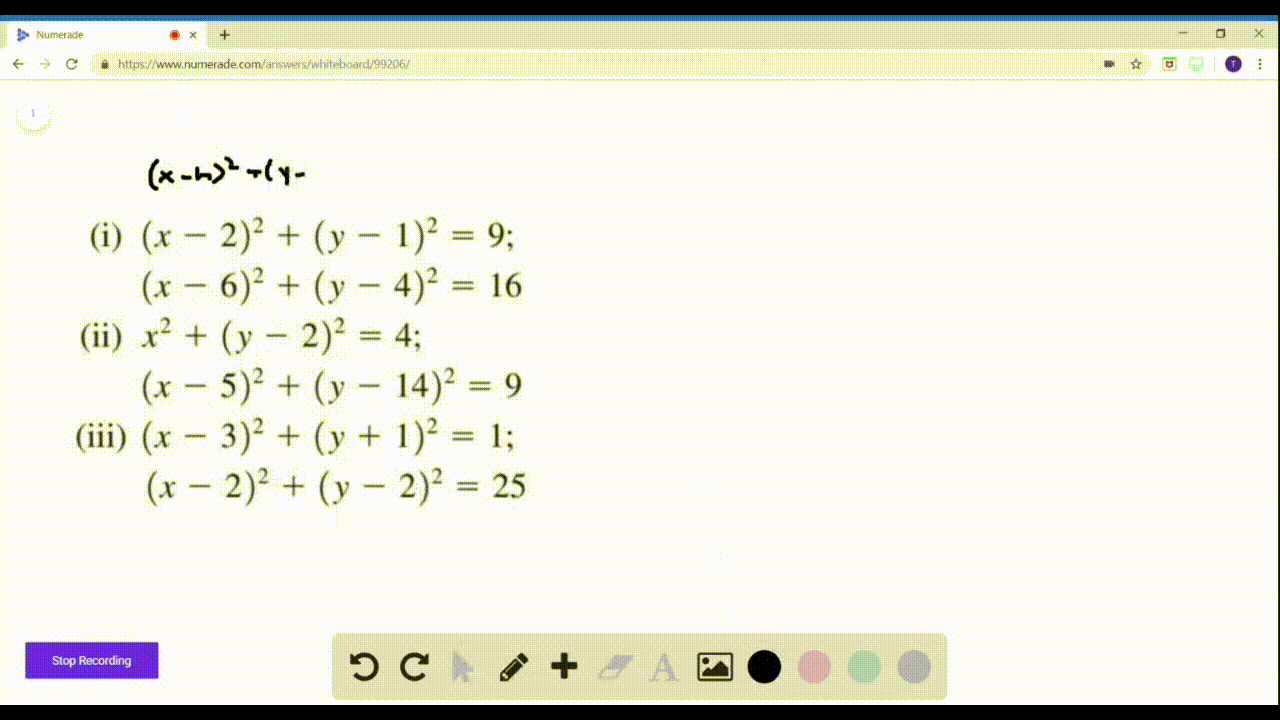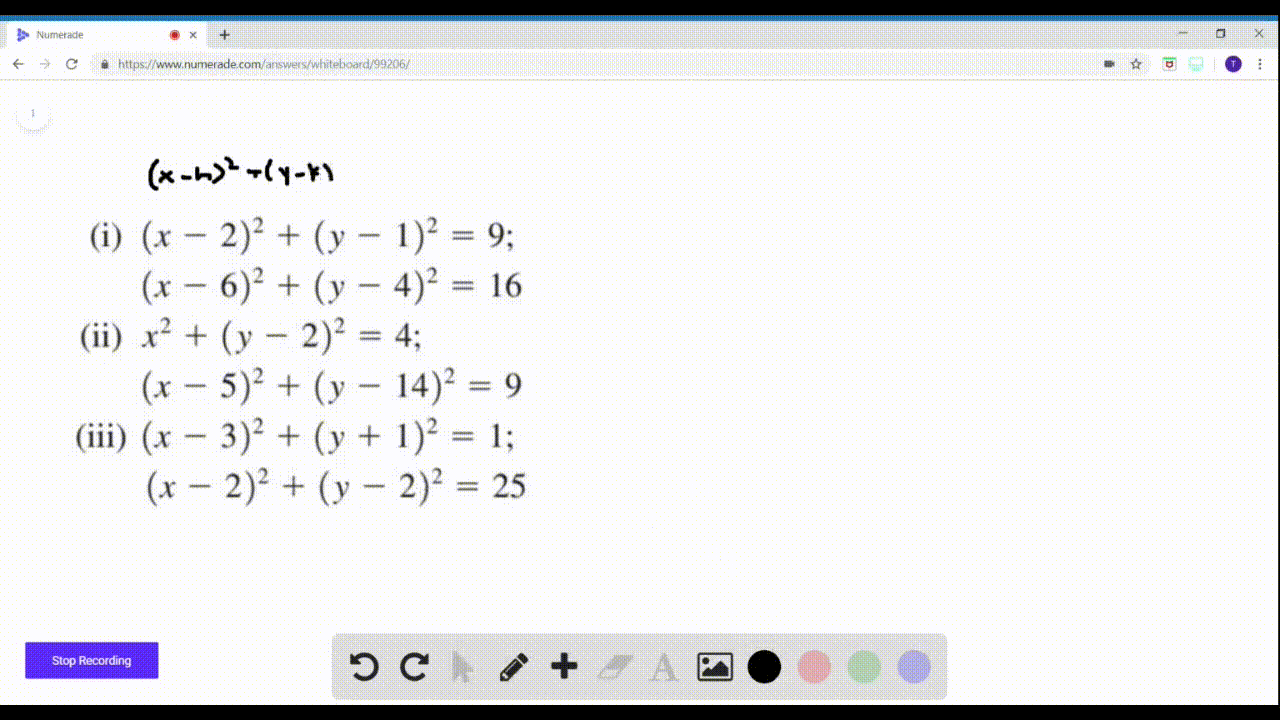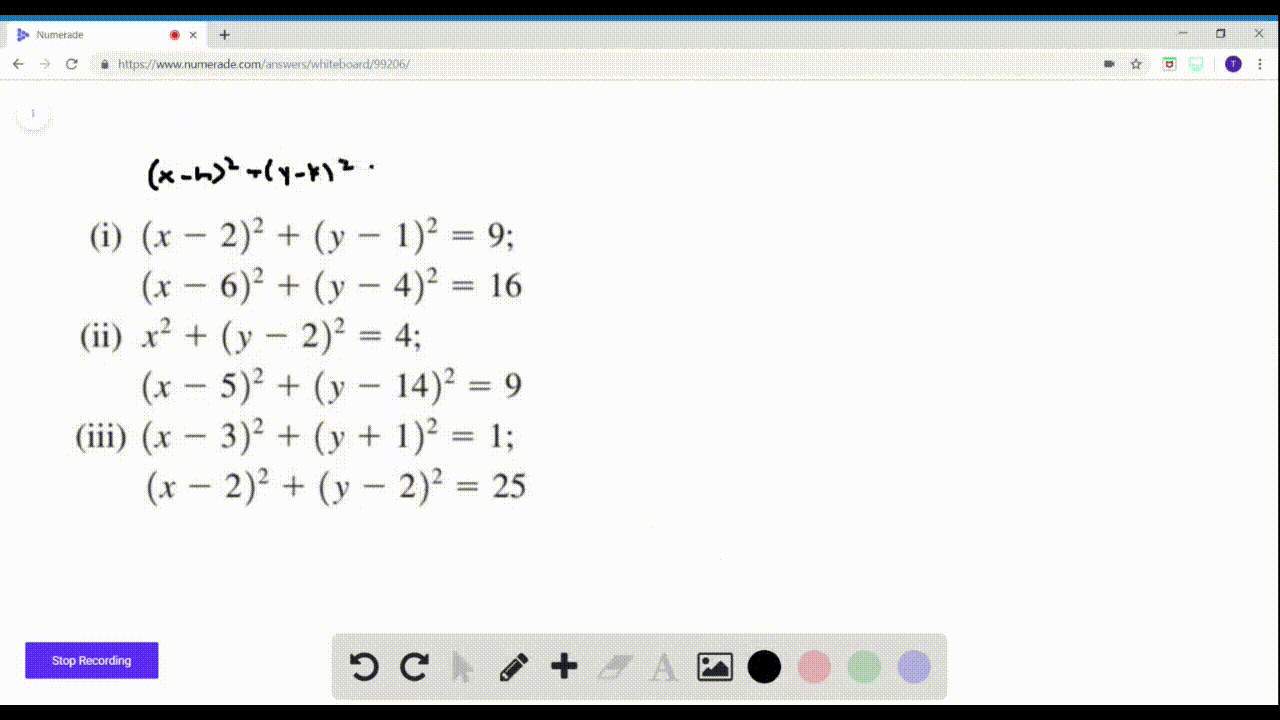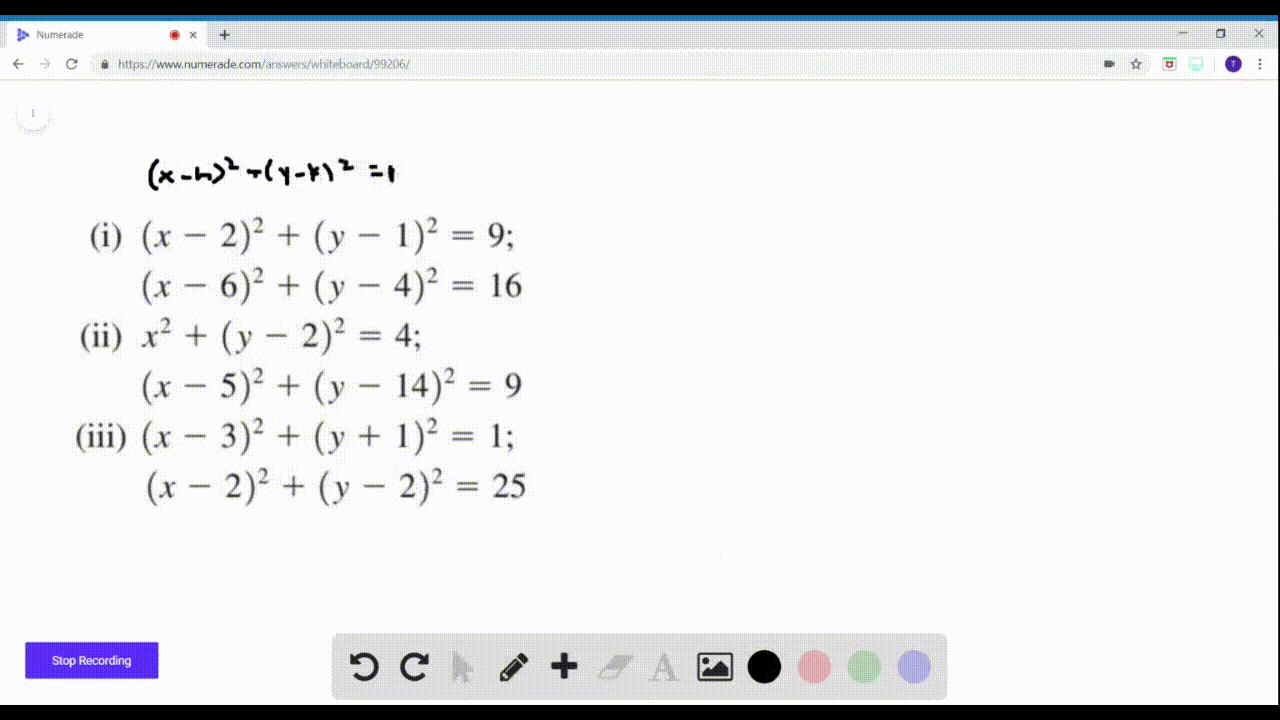


Solved Discover Circle Point Or Empty Set Com



The Centre And Radius Of The Circle X 2 Y 2 25 Is
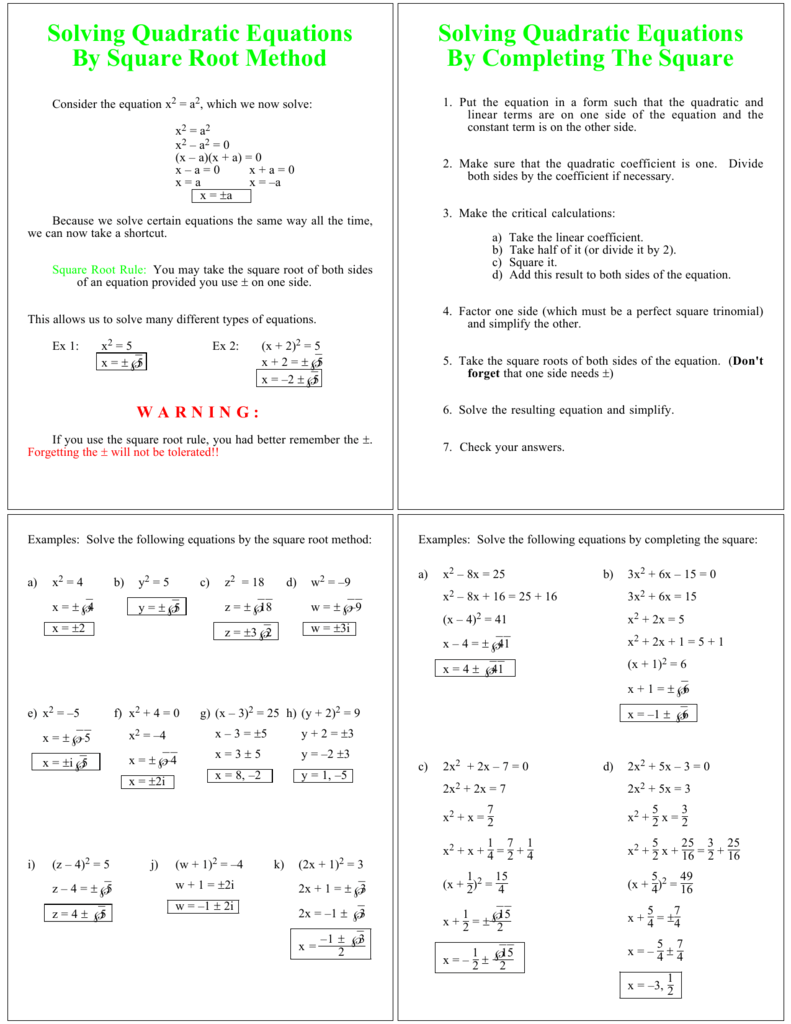


Solving Quadratic Equations By Square Root Method By
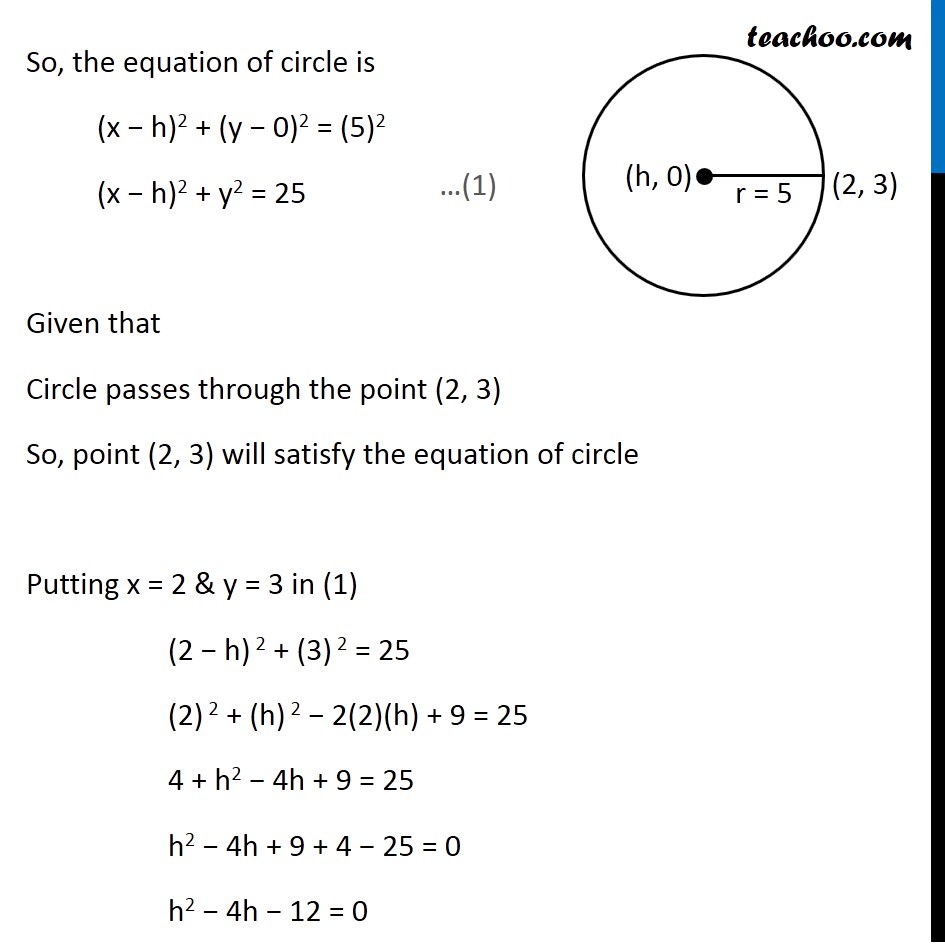


Ex 11 1 12 Find The Equation Of Circle With Radius 5 Whose Center



Graph X 2 Y 2 4 Youtube
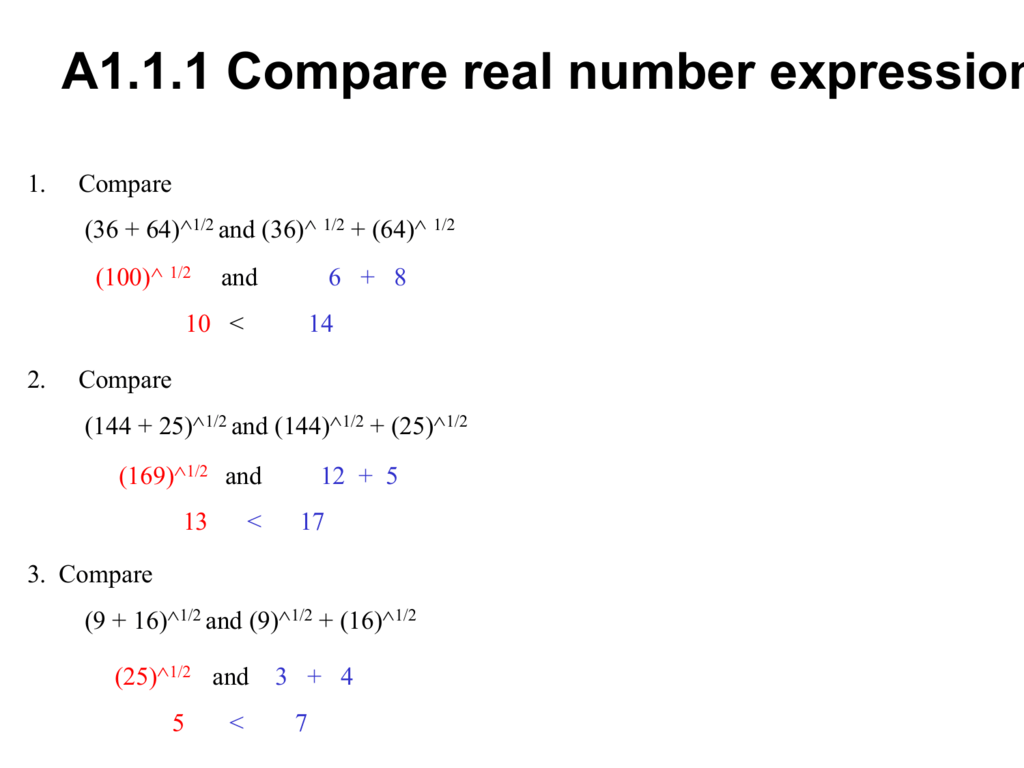


X 2
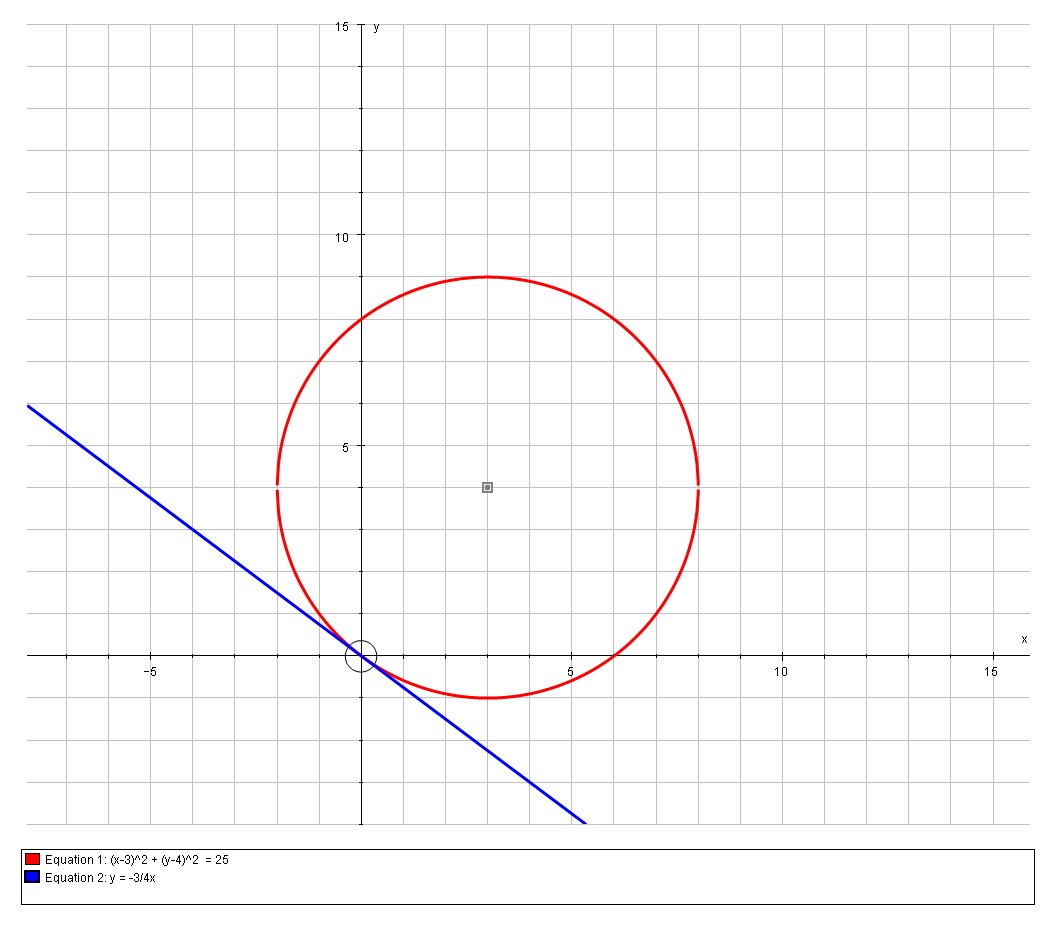


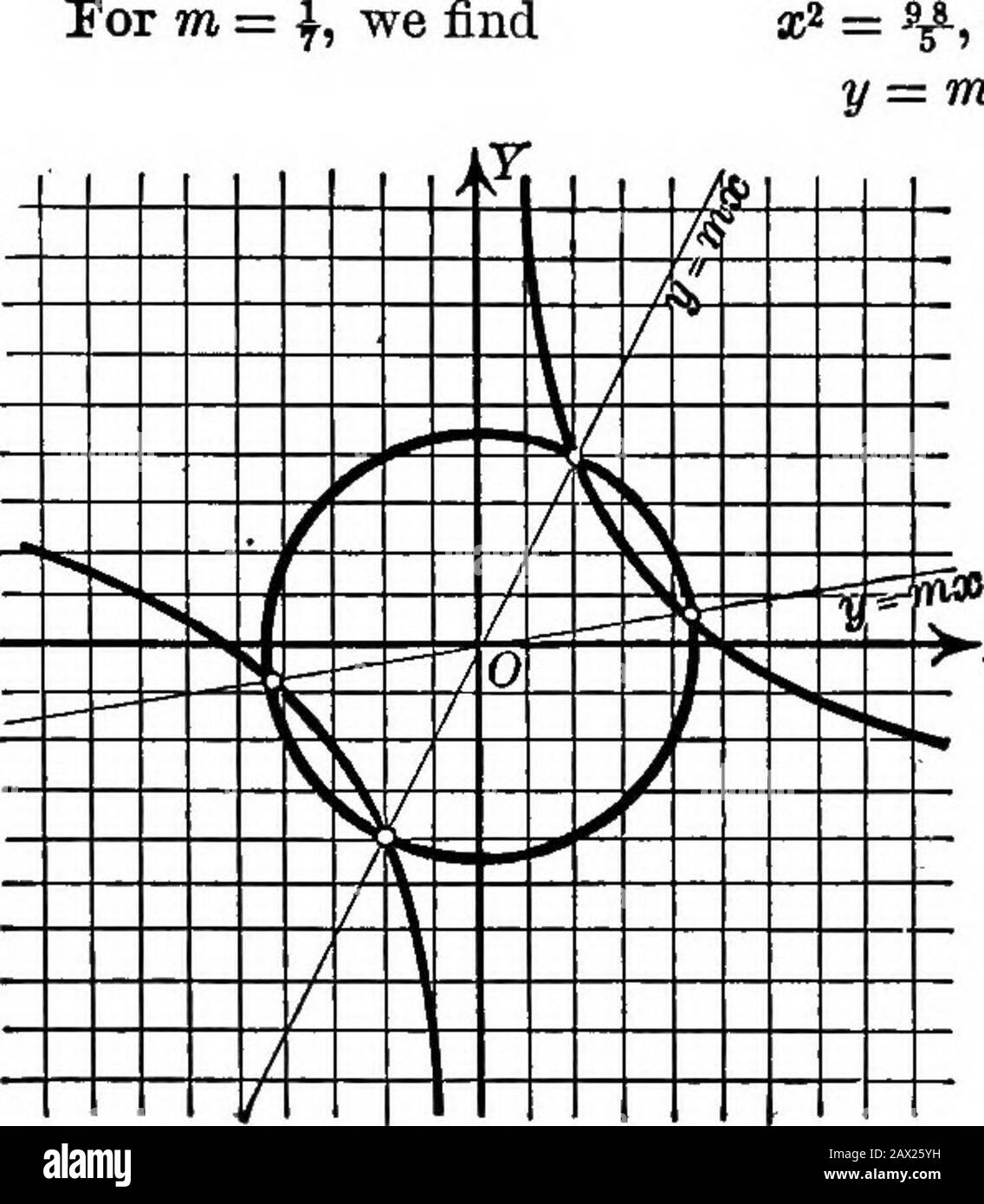
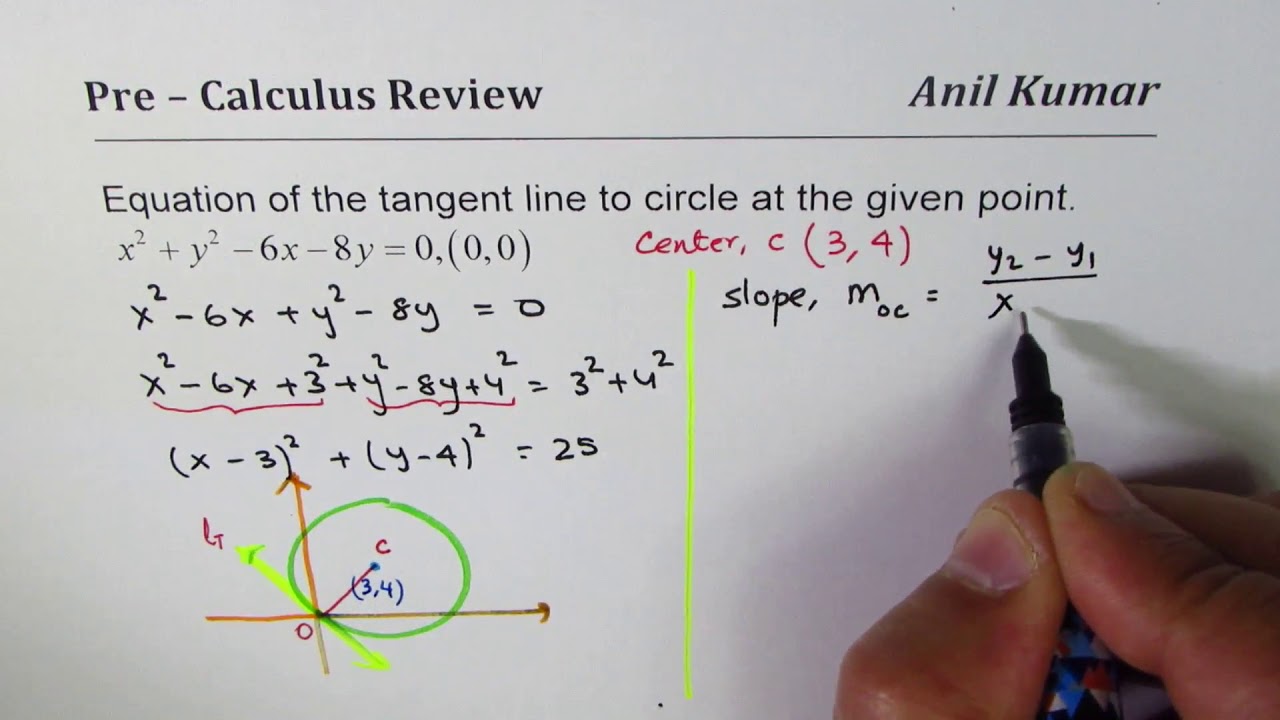
How Do You Write An Equation Of The Line Tangent To X 2 Y 2 6x 8y 0 At The Point 0 0 Socratic
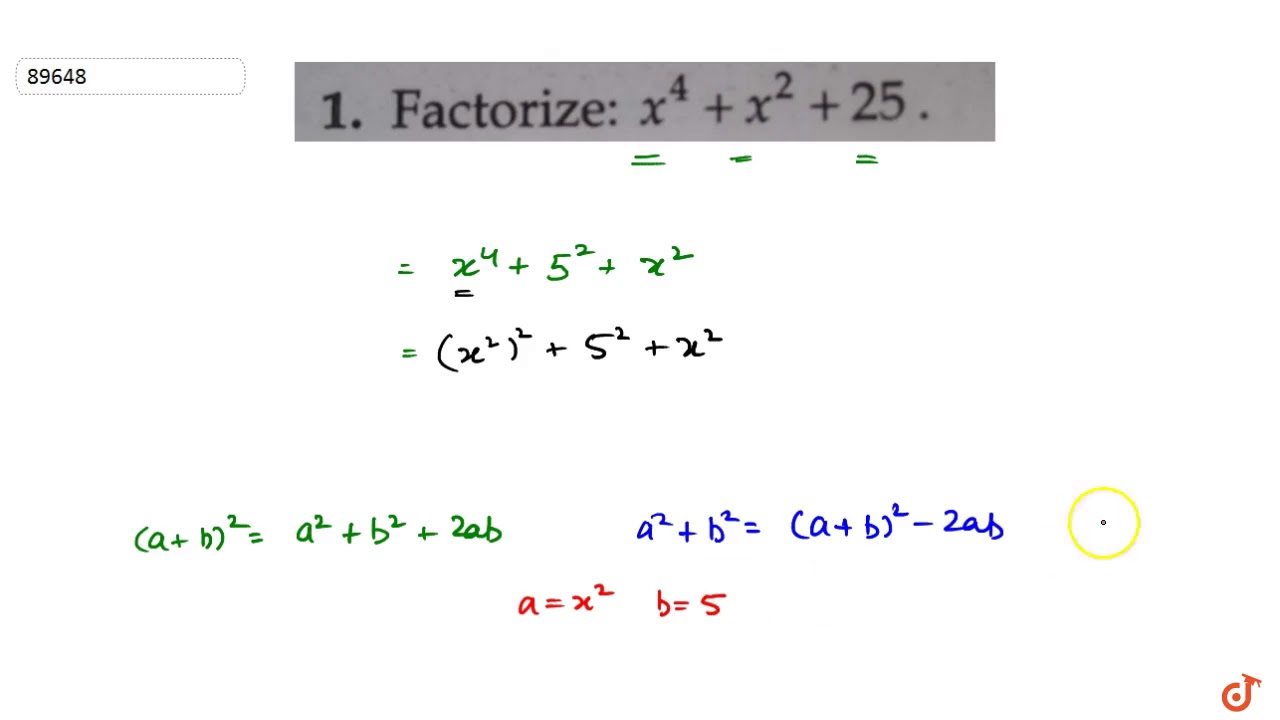


Factorise X 4 X 2 25 Youtube
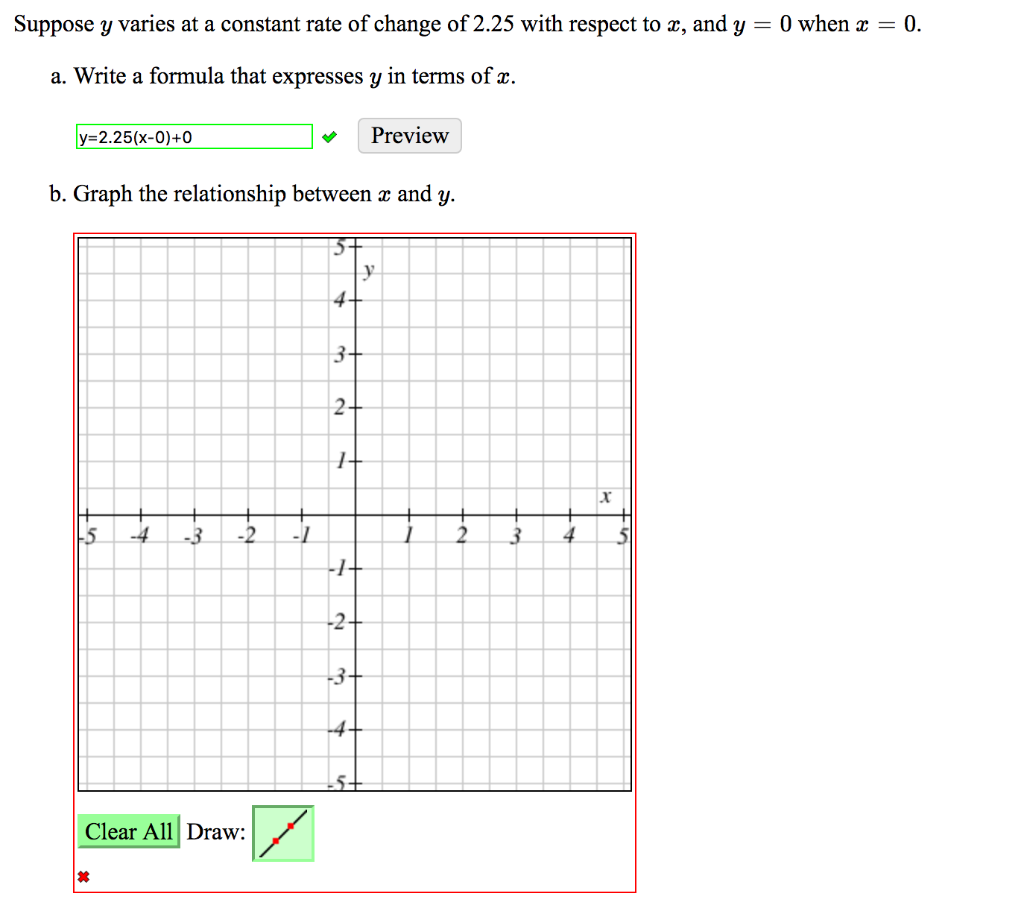


Solved Suppose Y Varies At A Constant Rate Of Change Of 3 Chegg Com
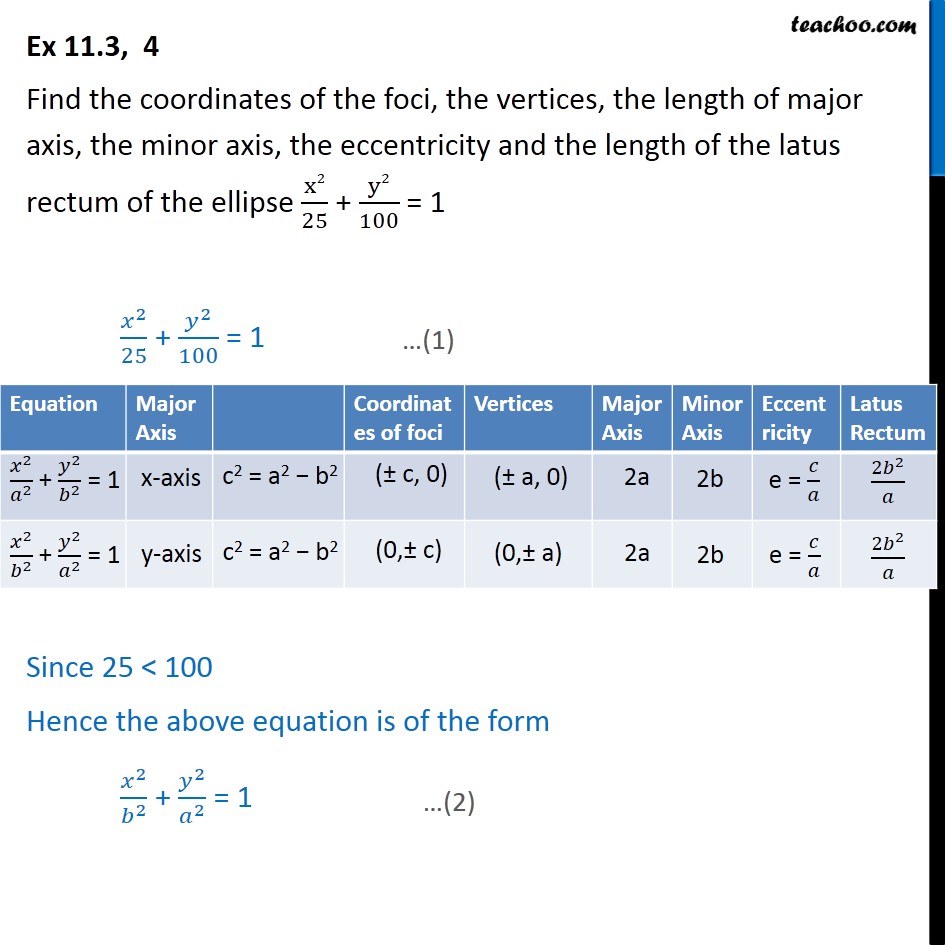


Ex 11 3 4 X2 25 Y2 100 1 Find Foci Latus Rectum Ex 11 3
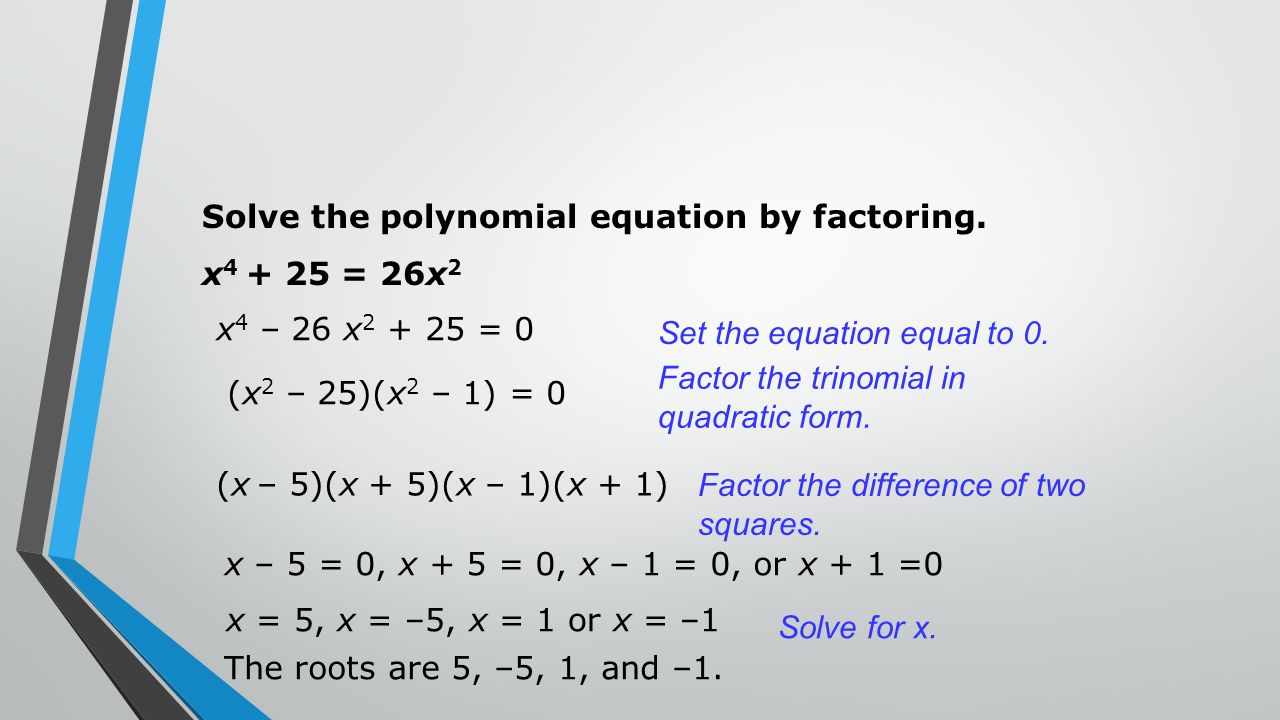


Circles Standard Form X H 2 Y K 2 R 2 Center H K Radius R Ppt Download
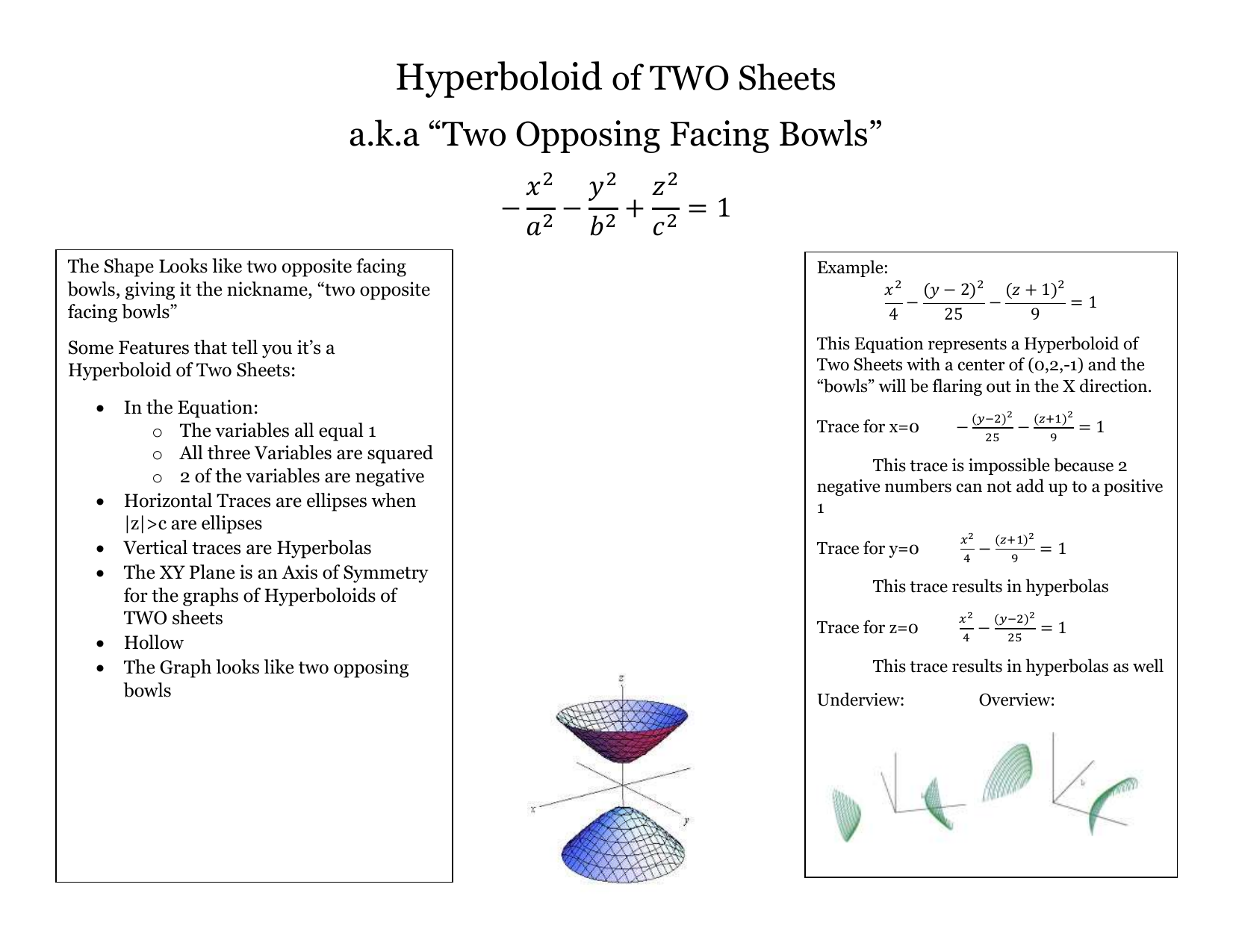


Hyperboloid Of Two Sheets
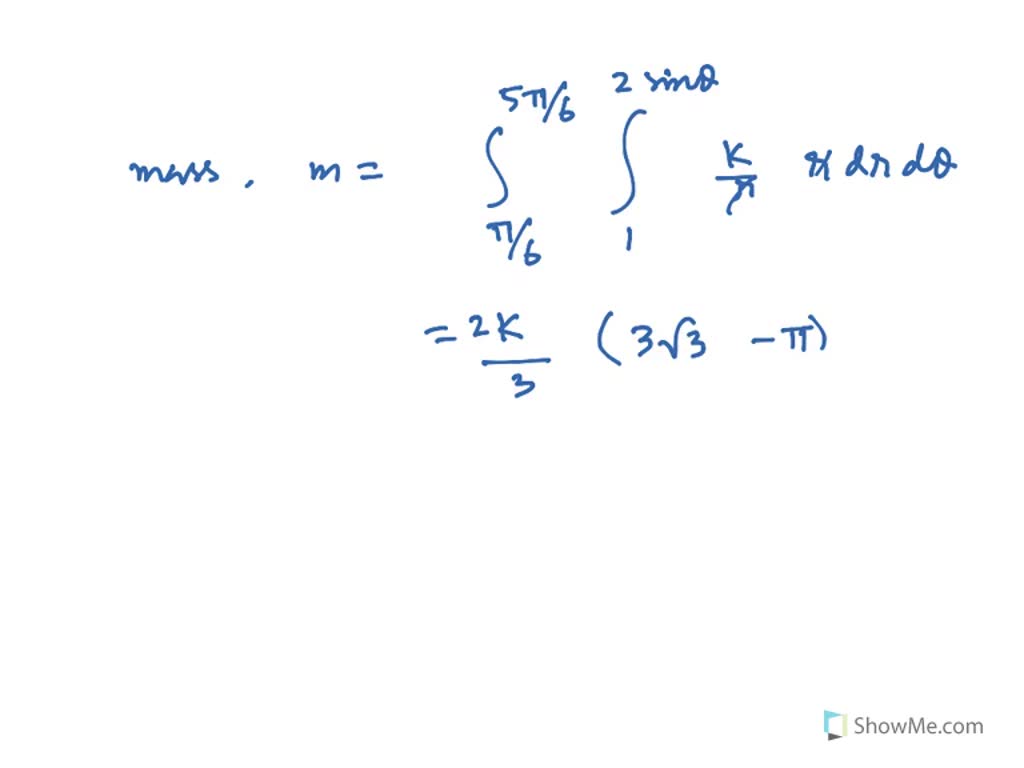


Solved A Lamina Occupies The Region Inside The Ci



画像をダウンロード 2x Y2 9x Y 5 中学 地理 プリント
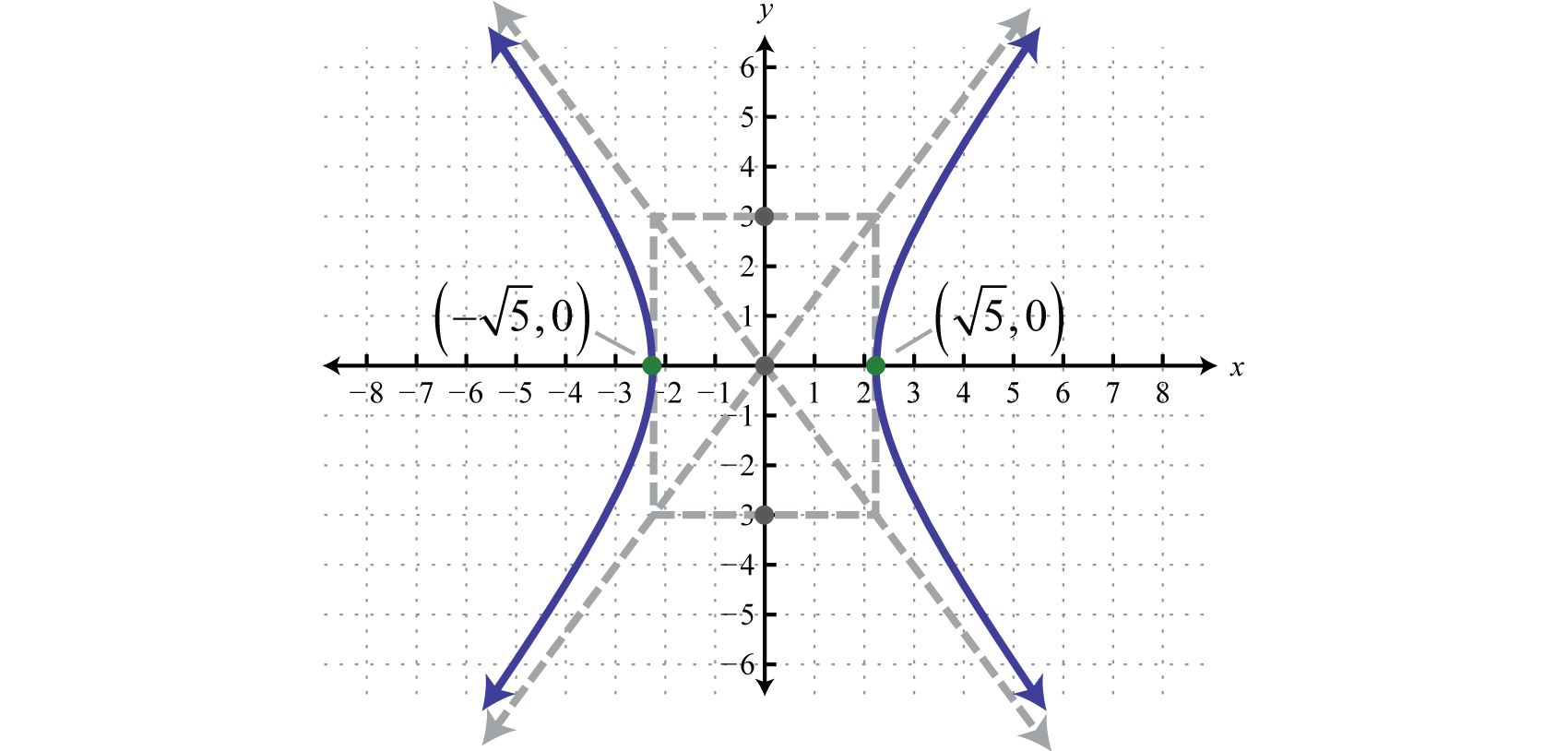


Hyperbolas
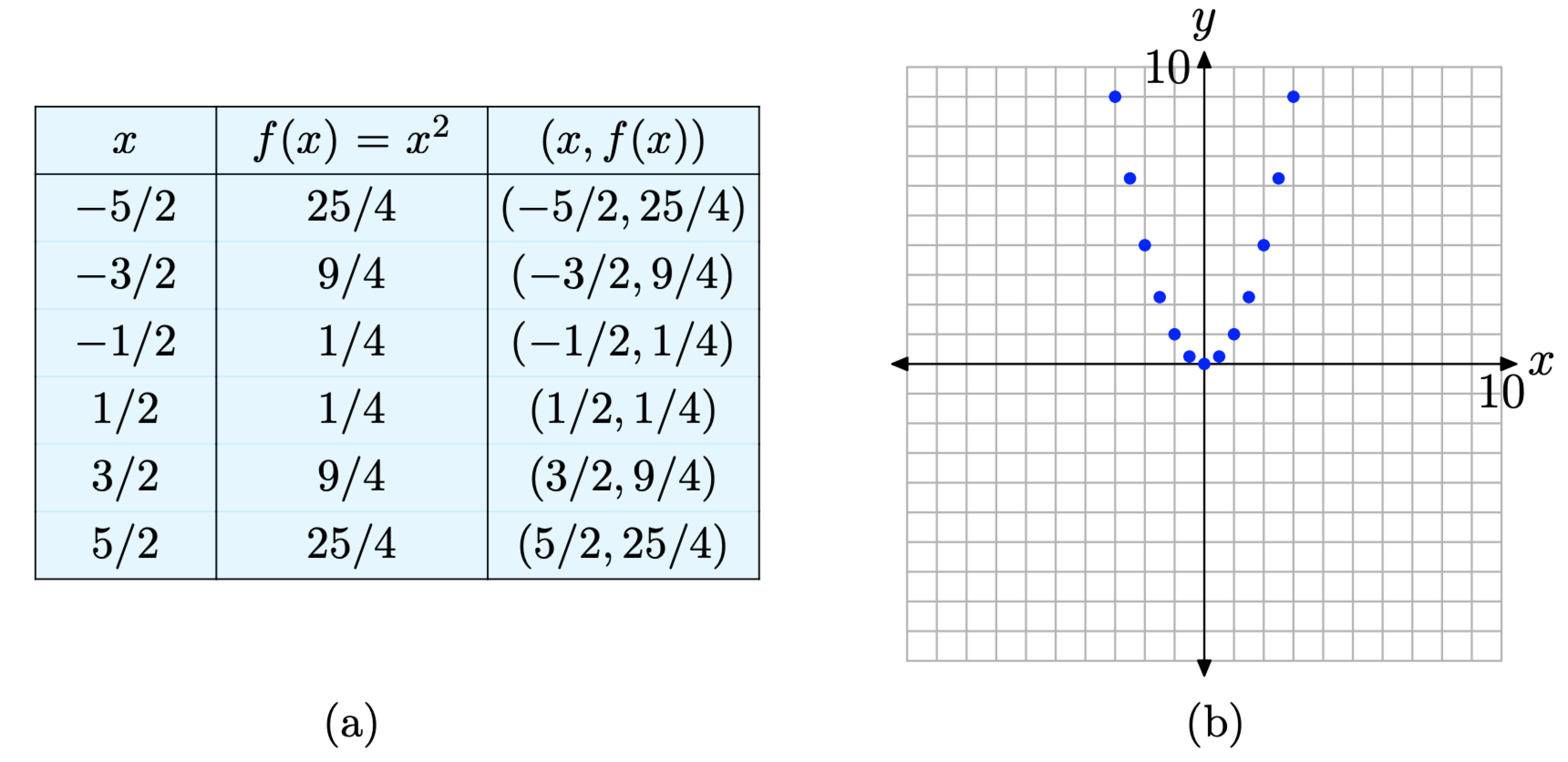


2 2 The Graph Of A Function Mathematics Libretexts



Geometry 10 7 Circles In The Coordinate Plane Ppt Download



Factorize I 25x 2 Y 2 Ii 4 25x 2 Iii X Y 2 1



How Do You Find The Integral Of X Sqrt 25 X 2 Socratic
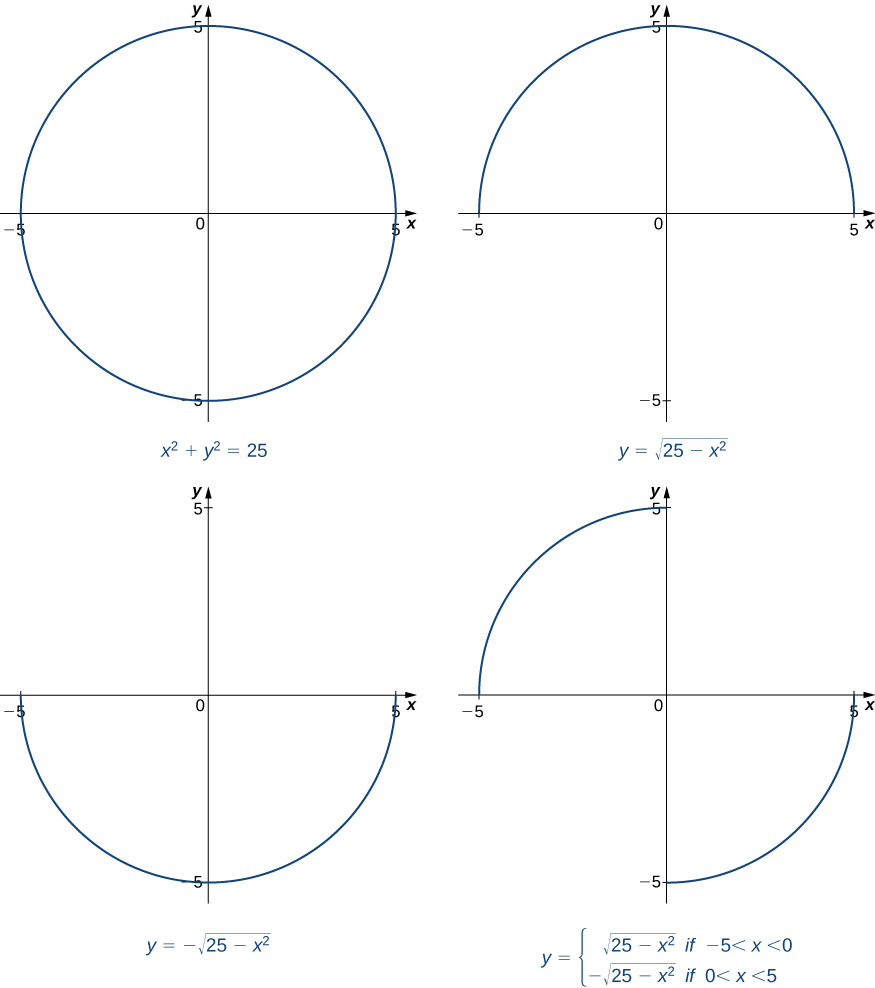


3 8 Implicit Differentiation Calculus Volume 1



Using The Average Method Of Solving Quadratic Equations Ii Math Inic



If R X Y X Y In W X 2 Y 2 25 Then Find The Domain An



The Equations Of The Tangents To The Circle X 2 Y 2 25 With Slop



Example 3 Find Centre Radius Of Circle X2 Y2 8x Examples
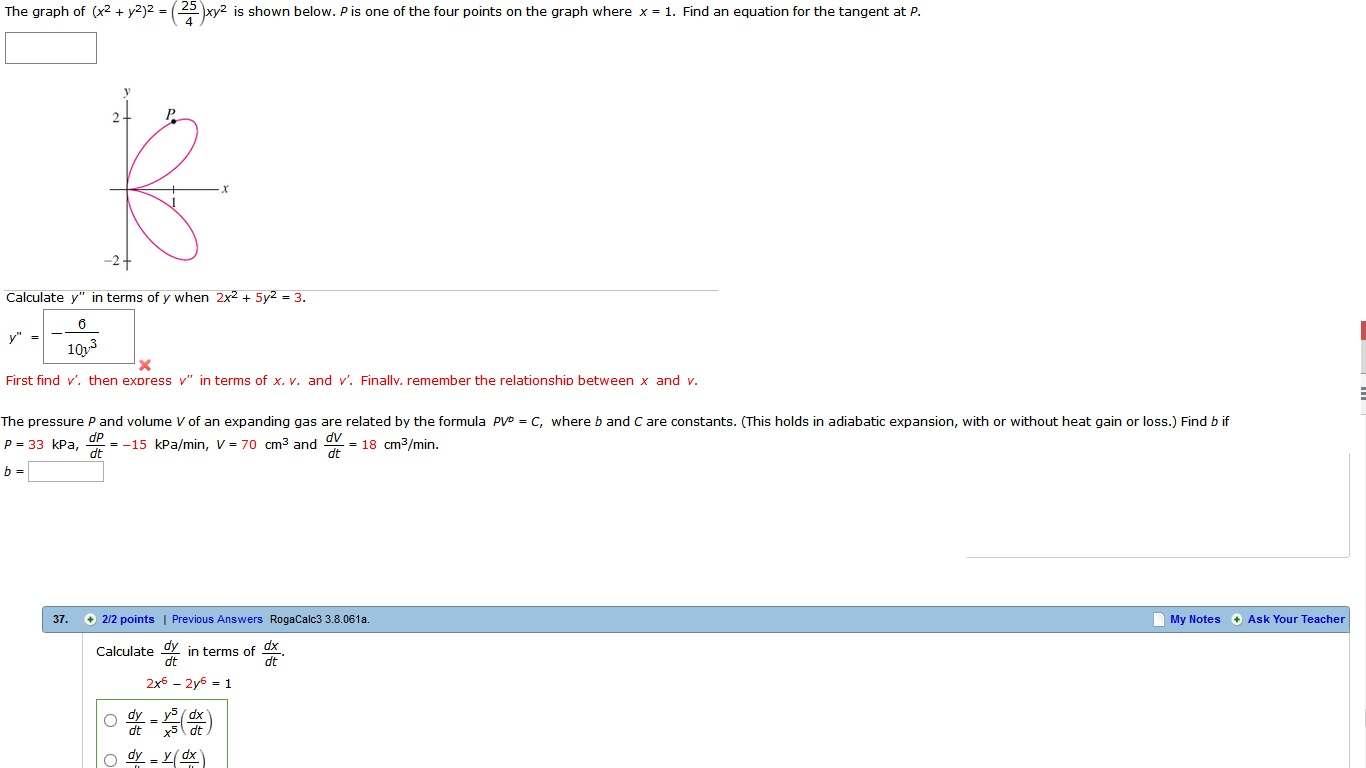


Solved The Graph Of X 2 Y 2 2 25 4 Xy 2 Is Shown B Chegg Com



How To Find The Equations Of The Asymptotes Of A Hyperbola


What Are The Points On The Curve X 4 Y 25 1 At Which The Tangents Are Parallel To X Axis Quora



Graphing Parabolas



Quadratic Systems A Line And A Circle Video Khan Academy


Writing Standard Equation Of A Circle Analytic Geometry Video Khan Academy



X 2 X 25 10x x Factorise Brainly In



Circles



How Do You Find An Equation For The Line Tangent To The Circle X 2 Y 2 25 At The Point 3 4 Socratic


Help With Quadratic Simultaneous Equation Gcse



Conic Section From Expanded Equation Hyperbola Video Khan Academy
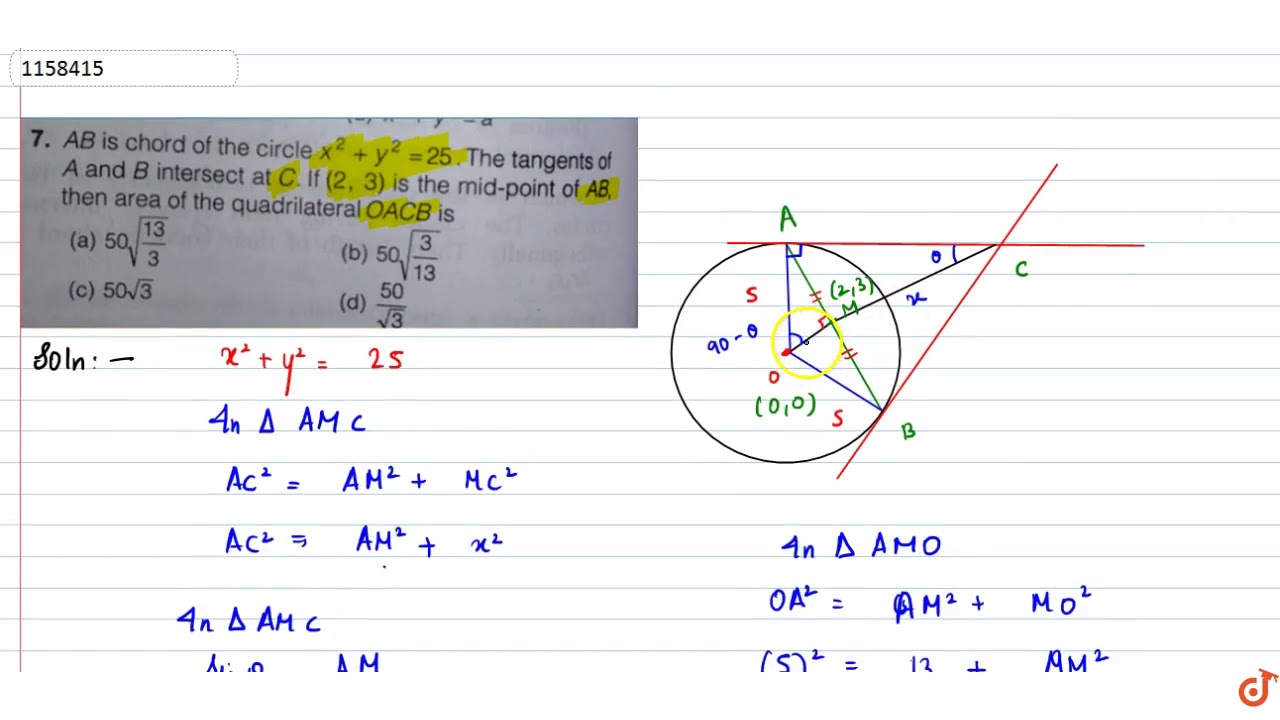


Ab Is A Chord Of The Circle X 2 Y 2 25 The Tangents Of A And B Intersect At C If 2 3 I Youtube



Conic Sections
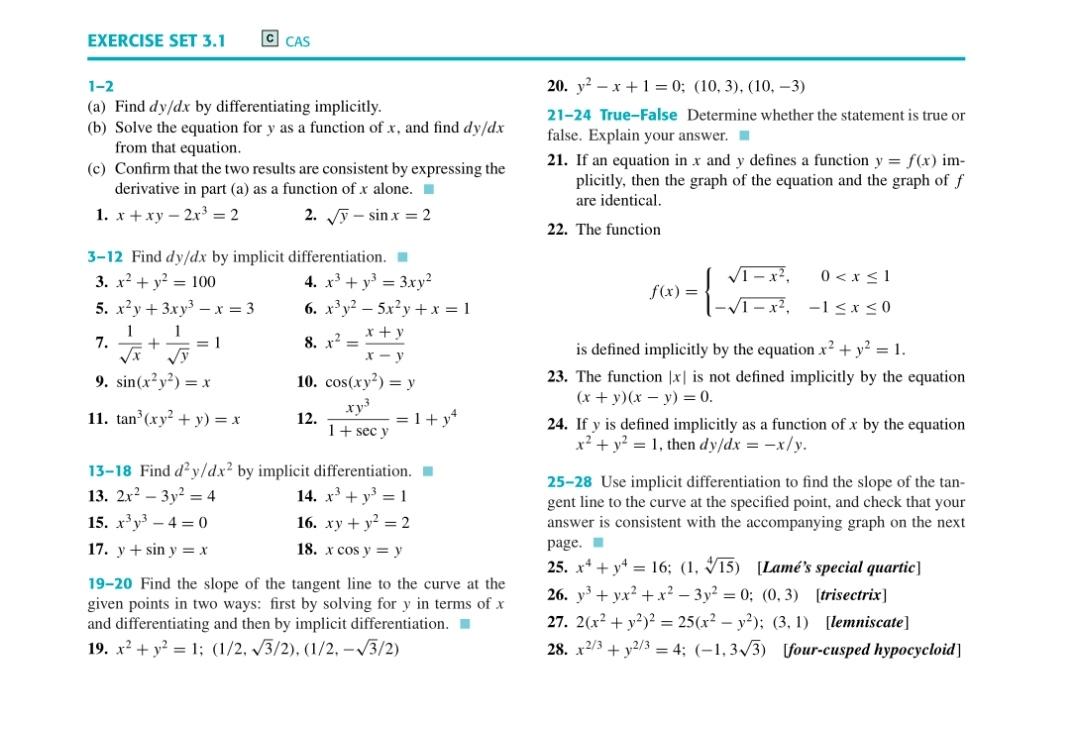


Solved Exercise Set 3 1 C Cas 1 2 A Find Dy Dx By Diffe Chegg Com
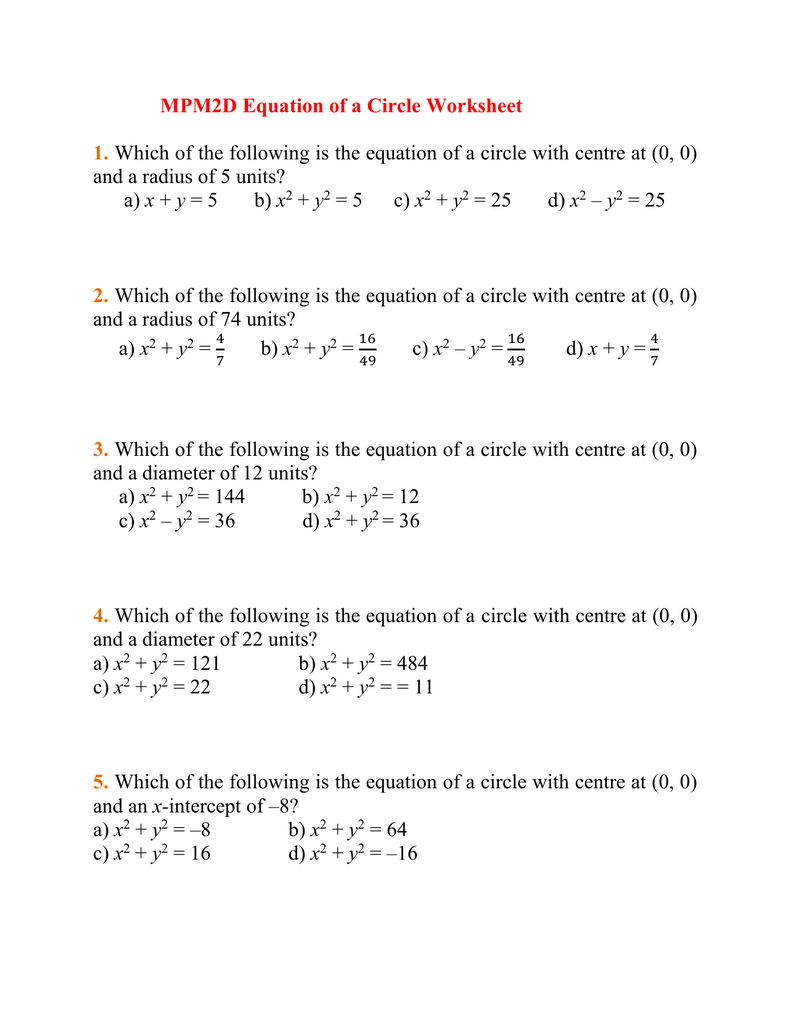


Equations Of Circles Worksheet Nidecmege
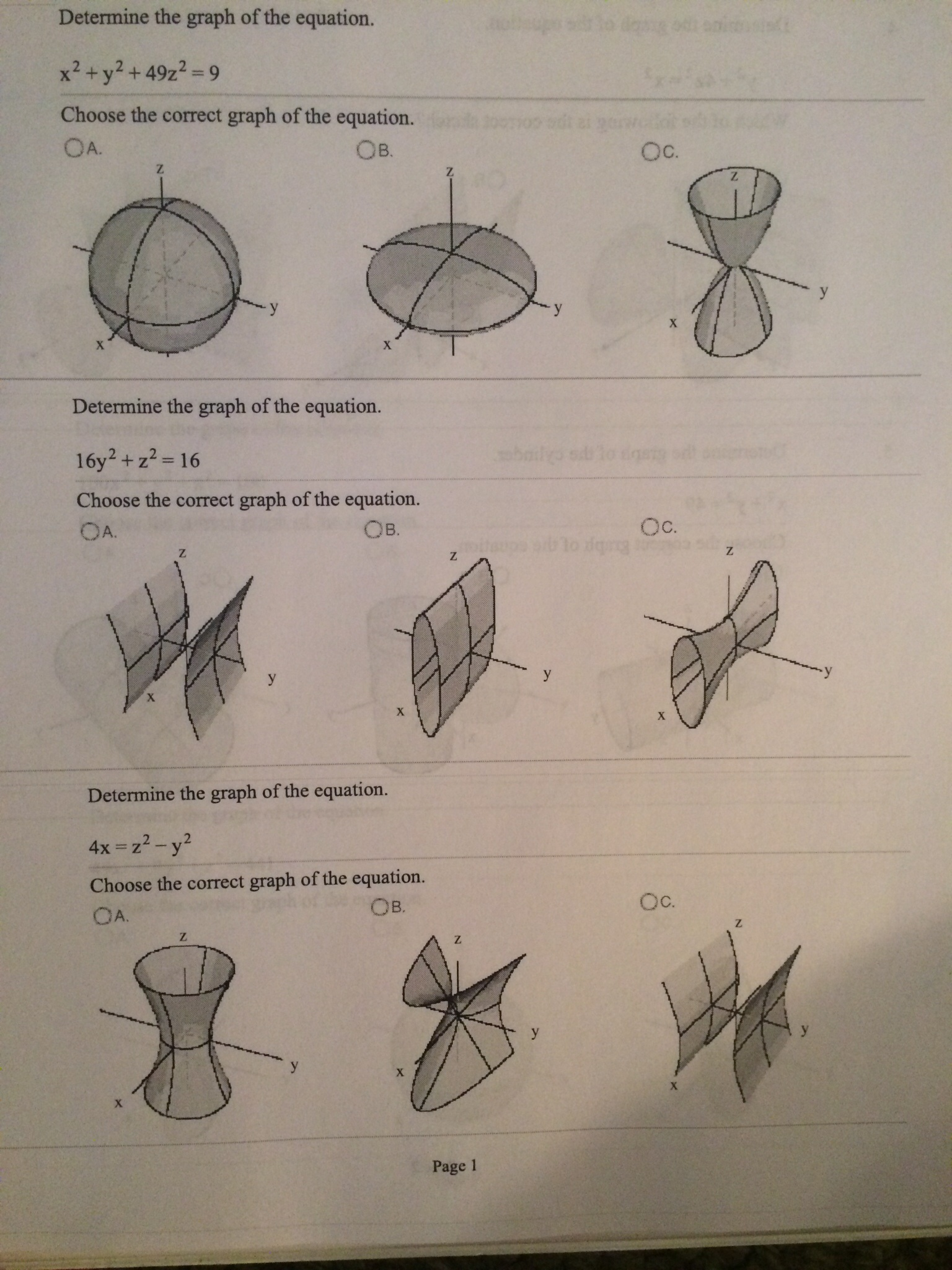


Solved Determine The Graph Of The Equation X2 Y2 49z Chegg Com



Find The Value Of X In The Triangle Abc Mathematics Stack Exchange
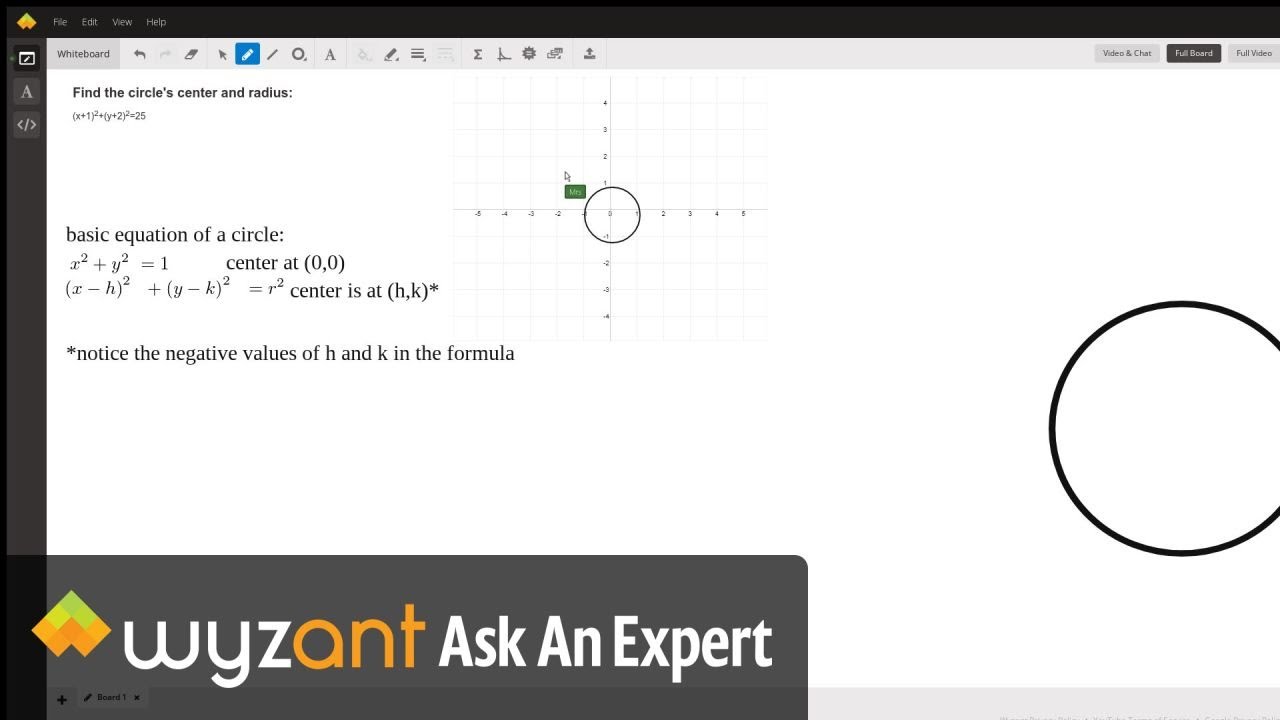


Find The Circle S Center And Radius Wyzant Ask An Expert
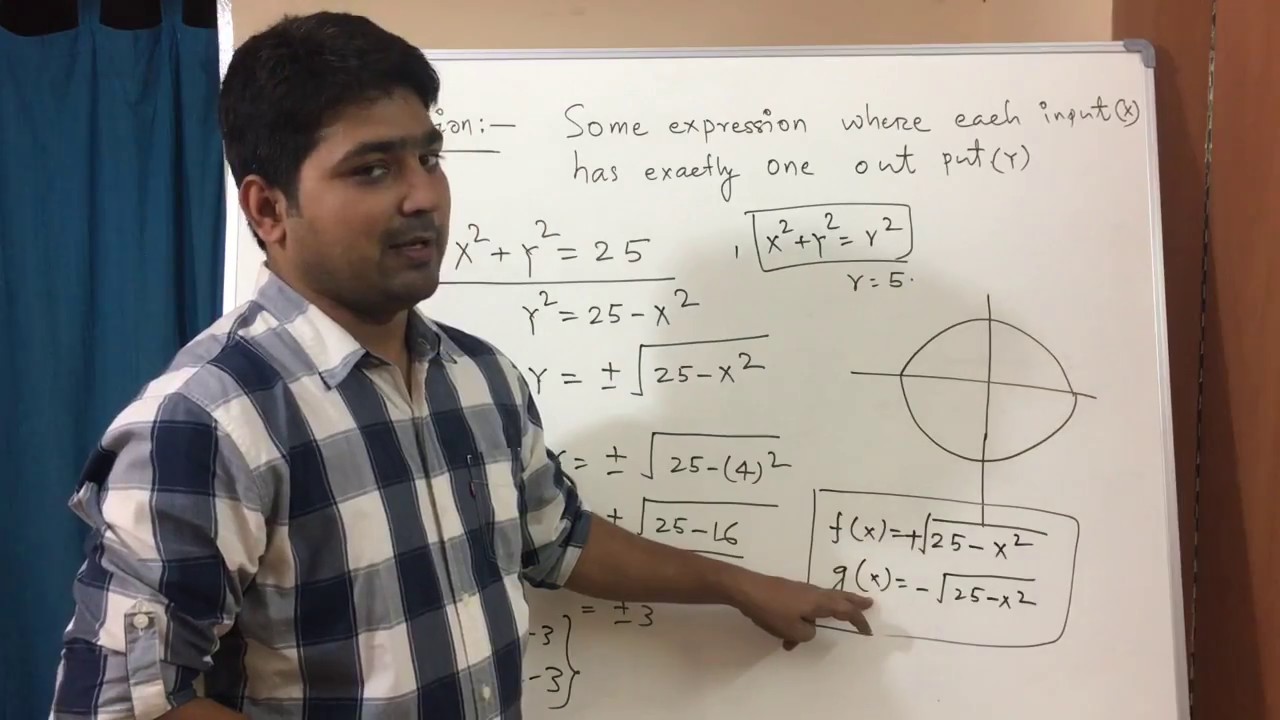


X Square Plus Y Square Equal To 25 Is It Function Understand Graphically In Hindi Youtube



No comments:
Post a Comment